
FIT2.3.3. Algebraic Extensions
Field Theory: We define an algebraic extension of a field F and show that successive algebraic extensions are also algebraic. This gives a useful criterion for checking algberaic elements. We finish with algebraic closures.
From playlist Abstract Algebra

Teena Gerhardt - 3/3 Algebraic K-theory and Trace Methods
Algebraic K-theory is an invariant of rings and ring spectra which illustrates a fascinating interplay between algebra and topology. Defined using topological tools, this invariant has important applications to algebraic geometry, number theory, and geometric topology. One fruitful approac
From playlist Summer School 2020: Motivic, Equivariant and Non-commutative Homotopy Theory

Teena Gerhardt - 1/3 Algebraic K-theory and Trace Methods
Algebraic K-theory is an invariant of rings and ring spectra which illustrates a fascinating interplay between algebra and topology. Defined using topological tools, this invariant has important applications to algebraic geometry, number theory, and geometric topology. One fruitful approac
From playlist Summer School 2020: Motivic, Equivariant and Non-commutative Homotopy Theory

Algebraic topology: Introduction
This lecture is part of an online course on algebraic topology. This is an introductory lecture, where we give a quick overview of some of the invariants of algebraic topology (homotopy groups, homology groups, K theory, and cobordism). The book "algebraic topology" by Allen Hatcher men
From playlist Algebraic topology

Guoliang Yu: Quantitative operator K-theory and its applications
Abstract: In this lecture, I will give an introduction to quantitative operator K-theory and apply it to compute K-theory of operator algebras which naturally arise from geometry. I will discuss applications to asymptotic behavior of positive scalar curvature when the dimension of the mani
From playlist HIM Lectures: Trimester Program "K-Theory and Related Fields"

Field Theory - Algebraically Closed Fields - Lecture 9
In this video we define what an algebraically closed field and assert without proof that they exist. We also explain why if you can find a single root for any polynomial, then you can find them all.
From playlist Field Theory
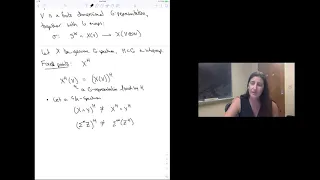
Teena Gerhardt - 2/3 Algebraic K-theory and Trace Methods
Algebraic K-theory is an invariant of rings and ring spectra which illustrates a fascinating interplay between algebra and topology. Defined using topological tools, this invariant has important applications to algebraic geometry, number theory, and geometric topology. One fruitful approac
From playlist Summer School 2020: Motivic, Equivariant and Non-commutative Homotopy Theory

Anna Marie Bohmann: Assembly in the Algebraic K-theory of Lawvere Theories
Talk by Anna Marie Bohmann in Global Noncommutative Geometry Seminar (Americas), https://globalncgseminar.org/talks/tba-30/, on April 29, 2022.
From playlist Global Noncommutative Geometry Seminar (Americas)

Haldun Özgür Bayindir : Adjoining roots to ring spectra and algebraic 𝐾-theory
CONFERENCE Recording during the thematic meeting : « Chromatic Homotopy, K-Theory and Functors» the January 24, 2023 at the Centre International de Rencontres Mathématiques (Marseille, France) Filmmaker: Jean Petit Find this video and other talks given by worldwide mathematicians on CIR
From playlist Topology

Simon Brain: The Gysin Sequence for Quantum Lens Spaces
This is a joint with Francesca Arici and Giovanni Landi. We construct an analogue of the Gysin sequence for circle bundles, now for q-deformed lens spaces in the sense of Vaksman-Soibelman. Our proof that the sequence is exact relies heavily on the non commutative APS index theory of Care
From playlist HIM Lectures: Trimester Program "Non-commutative Geometry and its Applications"

Nigel Higson: The Oka principle and Novodvorskii’s theorem
Talk by Jonathan Rosenberg in Global Noncommutative Geometry Seminar (Americas) http://www.math.wustl.edu/~xtang/NCG-Seminar.html on November 11, 2020.
From playlist Global Noncommutative Geometry Seminar (Americas)

Number theory Full Course [A to Z]
Number theory (or arithmetic or higher arithmetic in older usage) is a branch of pure #mathematics devoted primarily to the study of the integers and integer-valued functions. Number theorists study prime numbers as well as the properties of objects made out of integers (for example, ratio
From playlist Number Theory

In this video, we give an important motivation for studying Topological Cyclic Homology, so called "trace methods". Feel free to post comments and questions at our public forum at https://www.uni-muenster.de/TopologyQA/index.php?qa=tc-lecture Homepage with further information: https://w
From playlist Topological Cyclic Homology

Recent developments in non-commutative Iwasawa theory I - David Burns
David Burns March 25, 2011 For more videos, visit http://video.ias.edu
From playlist Mathematics

Bruno Klingler - 3/4 Tame Geometry and Hodge Theory
Hodge theory, as developed by Deligne and Griffiths, is the main tool for analyzing the geometry and arithmetic of complex algebraic varieties. It is an essential fact that at heart, Hodge theory is NOT algebraic. On the other hand, according to both the Hodge conjecture and the Grothendie
From playlist Bruno Klingler - Tame Geometry and Hodge Theory

Rufus Willett: Decomposable C*-algebras and the UCT
Talk by Rufus Willett in in Global Noncommutative Geometry Seminar (Americas) https://globalncgseminar.org/talks/tba-25/ on March 11, 2022.
From playlist Global Noncommutative Geometry Seminar (Americas)

Alex Fok, Equvariant twisted KK-theory of noncompact Lie groups
Global Noncommutative Geometry Seminar(Asia-Pacific), Oct. 25, 2021
From playlist Global Noncommutative Geometry Seminar (Asia and Pacific)

Bruno Klingler - 2/4 Tame Geometry and Hodge Theory
Hodge theory, as developed by Deligne and Griffiths, is the main tool for analyzing the geometry and arithmetic of complex algebraic varieties. It is an essential fact that at heart, Hodge theory is NOT algebraic. On the other hand, according to both the Hodge conjecture and the Grothendie
From playlist Bruno Klingler - Tame Geometry and Hodge Theory

Georg Tamme: Differential algebraic K theory
The lecture was held within the framework of the Hausdorff Trimester Program: Non-commutative Geometry and its Applications and the Workshop: Number theory and non-commutative geometry 28.11.2014
From playlist HIM Lectures: Trimester Program "Non-commutative Geometry and its Applications"