Elementary mathematics | Number theory | Cardinal numbers
Natural number
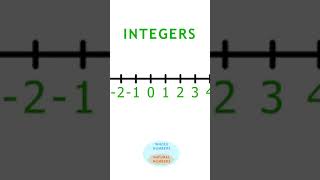
In mathematics, the natural numbers are those numbers used for counting (as in "there are six coins on the table") and ordering (as in "this is the third largest city in the country"). Numbers used for counting are called cardinal numbers, and numbers used for ordering are called ordinal numbers. Natural numbers are sometimes used as labels, known as nominal numbers, having none of the properties of numbers in a mathematical sense (e.g. sports jersey numbers). Some definitions, including the standard ISO 80000-2, begin the natural numbers with 0, corresponding to the non-negative integers 0, 1, 2, 3, ..., whereas others start with 1, corresponding to the positive integers 1, 2, 3, ... Texts that exclude zero from the natural numbers sometimes refer to the natural numbers together with zero as the whole numbers, while in other writings, that term is used instead for the integers (including negative integers). The natural numbers form a set. Many other number sets are built by successively extending the set of natural numbers: the integers, by including an additive identity 0 (if not yet in) and an additive inverse −n for each nonzero natural number n; the rational numbers, by including a multiplicative inverse for each nonzero integer n (and also the product of these inverses by integers); the real numbers by including the limits of (converging) Cauchy sequences of rationals; the complex numbers, by adjoining to the real numbers a square root of −1 (and also the sums and products thereof); and so on. This chain of extensions canonically embeds the natural numbers in the other number systems. Properties of the natural numbers, such as divisibility and the distribution of prime numbers, are studied in number theory. Problems concerning counting and ordering, such as partitioning and enumerations, are studied in combinatorics. In common language, particularly in primary school education, natural numbers may be called counting numbers to intuitively exclude the negative integers and zero, and also to contrast the discreteness of counting to the continuity of measurement—a hallmark characteristic of real numbers. (Wikipedia).