Infinitesimal

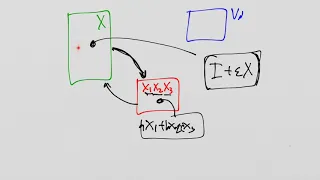
In mathematics, an infinitesimal number is a quantity that is closer to zero than any standard real number, but that is not zero. The word infinitesimal comes from a 17th-century Modern Latin coinage infinitesimus, which originally referred to the "infinity-th" item in a sequence. Infinitesimals do not exist in the standard real number system, but they do exist in other number systems, such as the surreal number system and the hyperreal number system, which can be thought of as the real numbers augmented with both infinitesimal and infinite quantities; the augmentations are the reciprocals of one another. Infinitesimal numbers were introduced in the development of calculus, in which the derivative was first conceived as a ratio of two infinitesimal quantities. This definition was not rigorously formalized. As calculus developed further, infinitesimals were replaced by limits, which can be calculated using the standard real numbers. Infinitesimals regained popularity in the 20th century with Abraham Robinson's development of nonstandard analysis and the hyperreal numbers, which, after centuries of controversy, showed that a formal treatment of infinitesimal calculus was possible. Following this, mathematicians developed surreal numbers, a related formalization of infinite and infinitesimal numbers that include both hyperreal cardinal and ordinal numbers, which is the largest ordered field. Vladimir Arnold wrote in 1990: Nowadays, when teaching analysis, it is not very popular to talk about infinitesimal quantities. Consequently, present-day students are not fully in command of this language. Nevertheless, it is still necessary to have command of it. The crucial insight for making infinitesimals feasible mathematical entities was that they could still retain certain properties such as angle or slope, even if these entities were infinitely small. Infinitesimals are a basic ingredient in calculus as developed by Leibniz, including the law of continuity and the transcendental law of homogeneity. In common speech, an infinitesimal object is an object that is smaller than any feasible measurement, but not zero in size—or, so small that it cannot be distinguished from zero by any available means. Hence, when used as an adjective in mathematics, infinitesimal means infinitely small, smaller than any standard real number. Infinitesimals are often compared to other infinitesimals of similar size, as in examining the derivative of a function. An infinite number of infinitesimals are summed to calculate an integral. The concept of infinitesimals was originally introduced around 1670 by either Nicolaus Mercator or Gottfried Wilhelm Leibniz. Archimedes used what eventually came to be known as the method of indivisibles in his work The Method of Mechanical Theorems to find areas of regions and volumes of solids. In his formal published treatises, Archimedes solved the same problem using the method of exhaustion. The 15th century saw the work of Nicholas of Cusa, further developed in the 17th century by Johannes Kepler, in particular, the calculation of the area of a circle by representing the latter as an infinite-sided polygon. Simon Stevin's work on the decimal representation of all numbers in the 16th century prepared the ground for the real continuum. Bonaventura Cavalieri's method of indivisibles led to an extension of the results of the classical authors. The method of indivisibles related to geometrical figures as being composed of entities of codimension 1. John Wallis's infinitesimals differed from indivisibles in that he would decompose geometrical figures into infinitely thin building blocks of the same dimension as the figure, preparing the ground for general methods of the integral calculus. He exploited an infinitesimal denoted 1/∞ in area calculations. The use of infinitesimals by Leibniz relied upon heuristic principles, such as the law of continuity: what succeeds for the finite numbers succeeds also for the infinite numbers and vice versa; and the transcendental law of homogeneity that specifies procedures for replacing expressions involving unassignable quantities, by expressions involving only assignable ones. The 18th century saw routine use of infinitesimals by mathematicians such as Leonhard Euler and Joseph-Louis Lagrange. Augustin-Louis Cauchy exploited infinitesimals both in defining continuity in his Cours d'Analyse, and in defining an early form of a Dirac delta function. As Cantor and Dedekind were developing more abstract versions of Stevin's continuum, Paul du Bois-Reymond wrote a series of papers on infinitesimal-enriched continua based on growth rates of functions. Du Bois-Reymond's work inspired both Émile Borel and Thoralf Skolem. Borel explicitly linked du Bois-Reymond's work to Cauchy's work on rates of growth of infinitesimals. Skolem developed the first non-standard models of arithmetic in 1934. A mathematical implementation of both the law of continuity and infinitesimals was achieved by Abraham Robinson in 1961, who developed nonstandard analysis based on earlier work by Edwin Hewitt in 1948 and Jerzy Łoś in 1955. The hyperreals implement an infinitesimal-enriched continuum and the transfer principle implements Leibniz's law of continuity. The standard part function implements Fermat's adequality. (Wikipedia).