
RNT1.4. Ideals and Quotient Rings
Ring Theory: We define ideals in rings as an analogue of normal subgroups in group theory. We give a correspondence between (two-sided) ideals and kernels of homomorphisms using quotient rings. We also state the First Isomorphism Theorem for Rings and give examples.
From playlist Abstract Algebra

Ideals in Ring Theory (Abstract Algebra)
An ideal of a ring is the similar to a normal subgroup of a group. Using an ideal, you can partition a ring into cosets, and these cosets form a new ring - a "factor ring." (Also called a "quotient ring.") After reviewing normal subgroups, we will show you *why* the definition of an ide
From playlist Abstract Algebra

Ring Theory: We define rings and give many examples. Items under consideration include commutativity and multiplicative inverses. Example include modular integers, square matrices, polynomial rings, quaternions, and adjoins of algebraic and transcendental numbers.
From playlist Abstract Algebra

Proof: Ideal of a Ring is Proper iff it has no Units | Abstract Algebra
An ideal of a ring with identity is proper if and only if the ideal contains no units of the ring. We prove this result in today's abstract algebra video lesson! Remember that an ideal, I, of a ring, R, is an additive subgroup of I such that for all r in R and x in I, r*x and x*r are also
From playlist Abstract Algebra

RNT2.1. Maximal Ideals and Fields
Ring Theory: We now consider special types of rings. In this part, we define maximal ideals and explore their relation to fields. In addition, we note three ways to construct fields.
From playlist Abstract Algebra

Definition of a Ring and Examples of Rings
Please Subscribe here, thank you!!! https://goo.gl/JQ8Nys Definition of a Ring and Examples of Rings - Definition of a Ring. - Definition of a commutative ring and a ring with identity. - Examples of Rings include: Z, Q, R, C under regular addition and multiplication The Ring of all n x
From playlist Abstract Algebra

Proof: Prime Ideal iff R/P is Integral Domain; Maximal iff R/M is Field
A very useful theorem in ring theory is the theorem that an ideal P is prime if and only if the quotient R/P is an integral domain (ID). Similarly, an ideal M is maximal if and only if R/M is a field. In this video, we prove both of these statements! Ring & Module Theory playlist: https:/
From playlist Ring & Module Theory

RNT1.2. Definition of Integral Domain
Ring Theory: We consider integral domains, which are commutative rings that contain no zero divisors. We show that this property is equivalent to a cancellation law for the ring. Finally we note some basic connections between integral domains and fields.
From playlist Abstract Algebra

Visual Group Theory: Lecture 7.4: Divisibility and factorization
Visual Group Theory: Lecture 7.4: Divisibility and factorization The ring of integers have a number of properties that we take for granted: every number can be factored uniquely into primes, and all pairs of numbers have a unique gcd and lcm. In this lecture, we investigate when this happ
From playlist Visual Group Theory
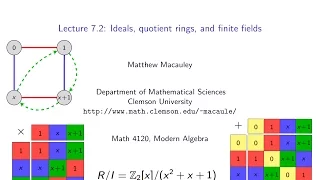
Visual Group Theory, Lecture 7.2: Ideals, quotient rings, and finite fields
Visual Group Theory, Lecture 7.2: Ideals, quotient rings, and finite fields A left (resp., right) ideal of a ring R is a subring that is invariant under left (resp., right) multiplication. Two-sided ideals are those that are both left and right ideals. This is the analogue of normal subgr
From playlist Visual Group Theory

Visual Group Theory, Lecture 7.1: Basic ring theory
Visual Group Theory, Lecture 7.1: Basic ring theory A ring is an abelian group (R,+) with a second binary operation, multiplication and the distributive law. Multiplication need not commute, nor need there be multiplicative inverses, so a ring is like a field but without these properties.
From playlist Visual Group Theory

Antonino Iannazzo, Queen Mary University of London
March 3, Antonino Iannazzo, Queen Mary University of London Differential Algebraic Geometry
From playlist Spring 2023 Online Kolchin Seminar in Differential Algebra

Introduction to the category of Adic spaces (Lecture 1) by Utsav Choudhury
PERFECTOID SPACES ORGANIZERS: Debargha Banerjee, Denis Benois, Chitrabhanu Chaudhuri, and Narasimha Kumar Cheraku DATE & TIME: 09 September 2019 to 20 September 2019 VENUE: Madhava Lecture Hall, ICTS, Bangalore Scientific committee: Jacques Tilouine (University of Paris, France) Eknath
From playlist Perfectoid Spaces 2019

A crash course in Algebraic Number Theory
A quick proof of the Prime Ideal Theorem (algebraic analog of the Prime Number Theorem) is presented. In algebraic number theory, the prime ideal theorem is the number field generalization of the prime number theorem. It provides an asymptotic formula for counting the number of prime idea
From playlist Number Theory

CTNT 2022 - 100 Years of Chebotarev Density (Lecture 1) - by Keith Conrad
This video is part of a mini-course on "100 Years of Chebotarev Density" that was taught during CTNT 2022, the Connecticut Summer School and Conference in Number Theory. More about CTNT: https://ctnt-summer.math.uconn.edu/
From playlist CTNT 2022 - 100 Years of Chebotarev Density (by Keith Conrad)

Commutative algebra 2 (Rings, ideals, modules)
This lecture is part of an online course on commutative algebra, following the book "Commutative algebra with a view toward algebraic geometry" by David Eisenbud. This lecture is a review of rings, ideals, and modules, where we give a few examples of non-commutative rings and rings without
From playlist Commutative algebra

Perfectoid spaces (Lecture 2) by Kiran Kedlaya
PERFECTOID SPACES ORGANIZERS: Debargha Banerjee, Denis Benois, Chitrabhanu Chaudhuri, and Narasimha Kumar Cheraku DATE & TIME: 09 September 2019 to 20 September 2019 VENUE: Madhava Lecture Hall, ICTS, Bangalore Scientific committee: Jacques Tilouine (University of Paris, France) Eknath
From playlist Perfectoid Spaces 2019
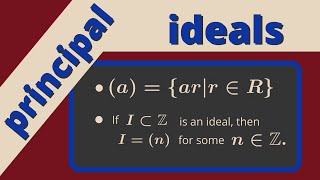
Abstract Algebra | Principal Ideals of a Ring
We define the notion of a principal ideal of a ring and give some examples. We also prove that all ideals of the integers are principal ideals, that is, the integers form a principal ideal domain (PID). http://www.michael-penn.net https://www.researchgate.net/profile/Michael_Penn5 http://
From playlist Abstract Algebra
Update on Tropical Schemes by Diane Maclagan
PROGRAM COMBINATORIAL ALGEBRAIC GEOMETRY: TROPICAL AND REAL (HYBRID) ORGANIZERS Arvind Ayyer (IISc, India), Madhusudan Manjunath (IITB, India) and Pranav Pandit (ICTS-TIFR, India) DATE: 27 June 2022 to 08 July 2022 VENUE: Madhava Lecture Hall and Online Algebraic geometry is the study of
From playlist Combinatorial Algebraic Geometry: Tropical and Real (HYBRID)
