
Abstract Algebra | The dihedral group
We present the group of symmetries of a regular n-gon, that is the dihedral group D_n. http://www.michael-penn.net http://www.randolphcollege.edu/mathematics/
From playlist Abstract Algebra

Symplectic geometry of surface group representations - William Goldman
Joint IAS/Princeton University Symplectic Geometry Seminar Topic: Symplectic geometry of surface group representations Speaker: William Goldman Affiliation: Member, School of Mathematics Date: February 28, 2022 If G is a Lie group whose adjoint representation preserves a nondegenerate sy
From playlist Mathematics
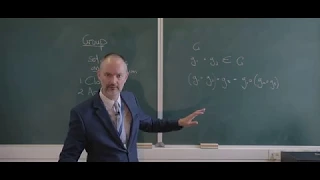
Definition of a group Lesson 24
In this video we take our first look at the definition of a group. It is basically a set of elements and the operation defined on them. If this set of elements and the operation defined on them obey the properties of closure and associativity, and if one of the elements is the identity el
From playlist Abstract algebra

What is a Group? | Abstract Algebra
Welcome to group theory! In today's lesson we'll be going over the definition of a group. We'll see the four group axioms in action with some examples, and some non-examples as well which violate the axioms and are thus not groups. In a fundamental way, groups are structures built from s
From playlist Abstract Algebra

Group theory 13: Dihedral groups
This lecture is part of an online mathematics course on group theory. It covers some basic properties of dihedral groups.
From playlist Group theory

Group theory 14: Sylow theorems
This lecture is part of an online mathematics course on group theory. It gives the proofs of the Sylow theorems about the Sylow p-subgroups: those of order the largest power of p dividing the order of a group. Correction: Yenan Wang pointed out that at 18:18 D4 should be D8, the dihedral
From playlist Group theory

Visual Group Theory, Lecture 1.6: The formal definition of a group
Visual Group Theory, Lecture 1.6: The formal definition of a group At last, after five lectures of building up our intuition of groups and numerous examples, we are ready to present the formal definition of a group. We conclude by proving several basic properties that are not built into t
From playlist Visual Group Theory
Group Theory II Symmetry Groups
Why are groups so popular? Well, in part it is because of their ability to characterise symmetries. This makes them a powerful tool in physics, where symmetry underlies our whole understanding of the fundamental forces. In this introduction to group theory, I explain the symmetry group of
From playlist Foundational Math

Stability conditions in symplectic topology – Ivan Smith – ICM2018
Geometry Invited Lecture 5.8 Stability conditions in symplectic topology Ivan Smith Abstract: We discuss potential (largely speculative) applications of Bridgeland’s theory of stability conditions to symplectic mapping class groups. ICM 2018 – International Congress of Mathematicians
From playlist Geometry

Homological mirror symmetry and symplectic mapping class groups - Nicholas Sheridan
Members' Seminar Topic: Homological mirror symmetry and symplectic mapping class groups Speaker: Nicholas Sheridan Affiliation: Princeton University; Member, School of Mathematics For more videos, visit http://video.ias.edu
From playlist Mathematics

Act globally, compute...points and localization - Tara Holm
Tara Holm Cornell University; von Neumann Fellow, School of Mathematics October 20, 2014 Localization is a topological technique that allows us to make global equivariant computations in terms of local data at the fixed points. For example, we may compute a global integral by summing inte
From playlist Mathematics

Ben Webster - Representation theory of symplectic singularities
Research lecture at the Worldwide Center of Mathematics
From playlist Center of Math Research: the Worldwide Lecture Seminar Series

What is a Group (Intuitively) - Group Theory 001
Here we begin to explore the mathematical objects that describe symmetry - Groups, and look at one particular example: the group of symmetries of a square 0:00 - Intro 0:25 - What is a group? 0:49 - Simple mathematical object example 1:49 - Symmetries 3:09 - A group is 3:20 - The group of
From playlist Summer of Math Exposition Youtube Videos

Maxence Mayrand: Hyperkähler structures on symplectic realizations of holomorphic Poisson surfaces
Recorded during the research school "Geometry and Dynamics of Foliations " the May 28, 2020 by the Centre International de Rencontres Mathématiques (Marseille, France) Filmmaker: Guillaume Hennenfent Find this video and other talks given by worldwide mathematicians on CIRM's Audiovisual Ma
From playlist VIRTUAL EVENT GEOMETRIC GROUP THEORY CONFERENCE

Brent Pym: Holomorphic Poisson structures - lecture 3
The notion of a Poisson manifold originated in mathematical physics, where it is used to describe the equations of motion of classical mechanical systems, but it is nowadays connected with many different parts of mathematics. A key feature of any Poisson manifold is that it carries a cano
From playlist Virtual Conference

Alessandra Sarti, Old and new on the symmetry groups of K3 surfaces
VaNTAGe Seminar, Feb 9, 2021
From playlist Arithmetic of K3 Surfaces

Rigidity and recurrence in symplectic dynamics - Matthias Schwarz
Members’ Seminar Topic: Rigidity and recurrence in symplectic dynamics Speaker: Matthias Schwarz, Universität Leipzig; Member, School of Mathematics Date: December 11, 2017 For more videos, please visit http://video.ias.edu
From playlist Mathematics

Symplectic Dynamics of Integrable Hamiltonian Systems - Alvaro Pelayo
Alvaro Pelayo Member, School of Mathematics April 4, 2011 I will start with a review the basic notions of Hamiltonian/symplectic vector field and of Hamiltonian/symplectic group action, and the classical structure theorems of Kostant, Atiyah, Guillemin-Sternberg and Delzant on Hamiltonian
From playlist Mathematics

Visual Group Theory, Lecture 5.6: The Sylow theorems
Visual Group Theory, Lecture 5.6: The Sylow theorems The three Sylow theorems help us understand the structure of non-abelian groups by placing strong restrictions on their p-subgroups (i.e., subgroups of prime power order). The first Sylow theorem says that for every p^k dividing |G|=p^n
From playlist Visual Group Theory
