
Axioms of Constructive Set Theory Explained
In this video we're going to discuss the various axiom schemes of constructive set theories and how they relate to type theory. I cover BCST, ECST, IKP, KPI, KP, CST, CZF, IZF, Mac Lane, Z and variants equi-consistent to ETCS from category theory, and then of course ZF and ZFC. The text I
From playlist Logic

Mark Bickford: Constructive Set Theory in Nuprl Type Theory
The lecture was held within the framework of the Hausdorff Trimester Program: Constructive Mathematics. Abstract: Aczel propsed CZF as a foundation for constructive mathematics and gave an interpretation of it in Martin-Löf type theory. He then extended the theory with the Regular Extens
From playlist Workshop: "Constructive Mathematics"

Henri Lombardi: A geometric theory for the constructive real number system and for o-minimal struct
Title: Henri Lombardi: A geometric theory for the constructive real number system and for o-minimal structures The lecture was held within the framework of the Hausdorff Trimester Program: Constructive Mathematics. Abstract: We work in a pure constructive context, minimalist, à la Bish
From playlist Workshop: "Constructive Mathematics"

Introduction to sets || Set theory Overview - Part 2
A set is the mathematical model for a collection of different things; a set contains elements or members, which can be mathematical objects of any kind: numbers, symbols, points in space, lines, other geometrical shapes, variables, or even other #sets. The #set with no element is the empty
From playlist Set Theory

Introduction to Set Theory (Discrete Mathematics)
Introduction to Set Theory (Discrete Mathematics) This is a basic introduction to set theory starting from the very beginning. This is typically found near the beginning of a discrete mathematics course in college or at the beginning of other advanced mathematics courses. ***************
From playlist Set Theory

We give some basic definitions and notions associated with sets. In particular, we describe sets via the "roster method", via a verbal description, and with set-builder notation. We also give an example of proving the equality of two sets. Please Subscribe: https://www.youtube.com/michael
From playlist Proof Writing

Introduction to sets || Set theory Overview - Part 1
A set is the mathematical model for a collection of different things; a set contains elements or members, which can be mathematical objects of any kind: numbers, symbols, points in space, lines, other geometrical shapes, variables, or even other #sets. The #set with no element is the empty
From playlist Set Theory

Giuseppe Rosolini: Categorical completions in constructive mathematics
The lecture was held within the framework of the Hausdorff Trimester Program: Constructive Mathematics. Abstract: There seems to be a very close connection between category theory and constructive mathematics which still is hard to make manifest, but which may be extremely useful to impr
From playlist Workshop: "Constructive Mathematics"
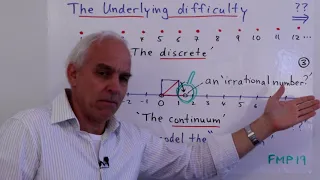
Modern "Set Theory" - is it a religious belief system? | Set Theory Math Foundations 250
Modern pure mathematics suffers from a uniform disinterest in examining the foundations of the subject carefully and objectively. The current belief system that "mathematics is based on set theory" is quite misguided, and in its current form represents an abdication of our responsibility t
From playlist Math Foundations

Constructive Type Theory and Homotopy - Steve Awodey
Steve Awodey Institute for Advanced Study December 3, 2010 In recent research it has become clear that there are fascinating connections between constructive mathematics, especially as formulated in the type theory of Martin-Löf, and homotopy theory, especially in the modern treatment in
From playlist Mathematics

On the Category of hSets - Bas Spitters
On the Category of hSets - Bas Spitters Bas Spitters Radboud University Nijmegen; Member, School of Mathematics April 3, 2013 For more videos, visit http://video.ias.edu
From playlist Mathematics

Univalent Foundations Seminar - Steve Awodey
Steve Awodey Carnegie Mellon University; Member, School of Mathematics November 19, 2012 For more videos, visit http://video.ias.edu
From playlist Mathematics

Homotopy Type Theory: what can logic do for homotopy theory? - Peter Lumsdaine
Peter Lumsdaine Homotopy Type Theory: what can logic do for homotopy theory? Institute for Advanced Study; Member, School of Mathematics October 4, 2013 For more videos, visit http://video.ias.edu
From playlist Mathematics

SHM - 16/01/15 - Constructivismes en mathématiques - Henri Lombardi
Henri Lombardi (LMB, Université de Franche-Comté), « Foundations of Constructive Analysis, Bishop, 1967 : une refondation des mathématiques, constructive, minimaliste et révolutionnaire »
From playlist Les constructivismes mathématiques - Séminaire d'Histoire des Mathématiques

Wolfram Physics Project: Working Session Tuesday, Nov. 2, 2021 [Topos Theory]
This is a Wolfram Physics Project working session about Topos Theory in the Wolfram Model. Originally livestreamed at: https://twitch.tv/stephen_wolfram Stay up-to-date on this project by visiting our website: http://wolfr.am/physics Check out the announcement post: http://wolfr.am/
From playlist Wolfram Physics Project Livestream Archive
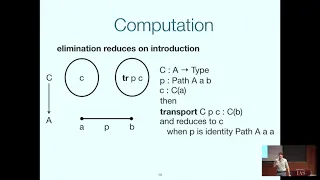
Univalence from a computer science point-of-view - Dan Licata
Vladimir Voevodsky Memorial Conference Topic: Univalence from a computer science point-of-view Speaker: Dan Licata Affiliation: Wesleyan University Date: September 14, 2018 For more video please visit http://video.ias.edu
From playlist Mathematics

Benedikt Ahrens - Univalent Foundations and the UniMath library - IPAM at UCLA
Recorded 13 February 2023. Benedikt Ahrens of Delft University of Technology presents "Univalent Foundations and the UniMath library" at IPAM's Machine Assisted Proofs Workshop. Abstract: Univalent Foundations (UF) were designed by Voevodsky as a foundation of mathematics that is "invarian
From playlist 2023 Machine Assisted Proofs Workshop

Yang-Mills for mathematicians (Lecture - 01) by Sourav Chatterjee
INFOSYS-ICTS RAMANUJAN LECTURES SOME OPEN QUESTIONS ABOUT SCALING LIMITS IN PROBABILITY THEORY SPEAKER Sourav Chatterjee (Stanford University, California, USA) DATE & TIME 14 January 2019 to 18 January 2019 VENUE Madhava Lecture Hall, ICTS campus GALLERY Lecture 1: Yang-Mills for mathemat
From playlist Infosys-ICTS Ramanujan Lectures

Review of set theory -- Proofs
This lecture is on Introduction to Higher Mathematics (Proofs). For more see http://calculus123.com.
From playlist Proofs