Incidence geometry | Conformal geometry | History of geometry | Euclidean plane geometry
Problem of Apollonius
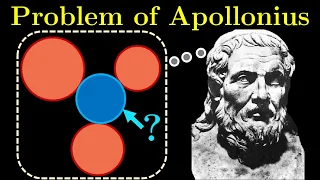
In Euclidean plane geometry, Apollonius's problem is to construct circles that are tangent to three given circles in a plane (Figure 1). Apollonius of Perga (c. 262 BC – c. 190 BC) posed and solved this famous problem in his work Ἐπαφαί (Epaphaí, "Tangencies"); this work has been lost, but a 4th-century AD report of his results by Pappus of Alexandria has survived. Three given circles generically have eight different circles that are tangent to them (Figure 2), a pair of solutions for each way to divide the three given circles in two subsets (there are 4 ways to divide a set of cardinality 3 in 2 parts). In the 16th century, Adriaan van Roomen solved the problem using intersecting hyperbolas, but this solution does not use only straightedge and compass constructions. François Viète found such a solution by exploiting limiting cases: any of the three given circles can be shrunk to zero radius (a point) or expanded to infinite radius (a line). Viète's approach, which uses simpler limiting cases to solve more complicated ones, is considered a plausible reconstruction of Apollonius' method. The method of van Roomen was simplified by Isaac Newton, who showed that Apollonius' problem is equivalent to finding a position from the differences of its distances to three known points. This has applications in navigation and positioning systems such as LORAN. Later mathematicians introduced algebraic methods, which transform a geometric problem into algebraic equations. These methods were simplified by exploiting symmetries inherent in the problem of Apollonius: for instance solution circles generically occur in pairs, with one solution enclosing the given circles that the other excludes (Figure 2). Joseph Diaz Gergonne used this symmetry to provide an elegant straightedge and compass solution, while other mathematicians used geometrical transformations such as reflection in a circle to simplify the configuration of the given circles. These developments provide a geometrical setting for algebraic methods (using Lie sphere geometry) and a classification of solutions according to 33 essentially different configurations of the given circles. Apollonius' problem has stimulated much further work. Generalizations to three dimensions—constructing a sphere tangent to four given spheres—and beyond have been studied. The configuration of three mutually tangent circles has received particular attention. René Descartes gave a formula relating the radii of the solution circles and the given circles, now known as Descartes' theorem. Solving Apollonius' problem iteratively in this case leads to the Apollonian gasket, which is one of the earliest fractals to be described in print, and is important in number theory via Ford circles and the Hardy–Littlewood circle method. (Wikipedia).