Articles containing proofs | Euclidean plane geometry | Euclidean symmetries | Group theory
Euclidean plane isometry
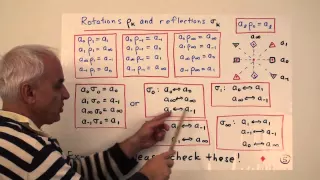
In geometry, a Euclidean plane isometry is an isometry of the Euclidean plane, or more informally, a way of transforming the plane that preserves geometrical properties such as length. There are four types: translations, rotations, reflections, and glide reflections (see below under classification of Euclidean plane isometries). The set of Euclidean plane isometries forms a group under composition: the Euclidean group in two dimensions. It is generated by reflections in lines, and every element of the Euclidean group is the composite of at most three distinct reflections. (Wikipedia).