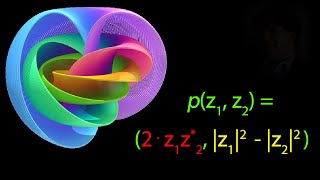Hopf fibration

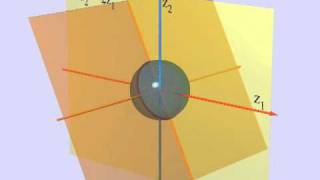
In the mathematical field of differential topology, the Hopf fibration (also known as the Hopf bundle or Hopf map) describes a 3-sphere (a hypersphere in four-dimensional space) in terms of circles and an ordinary sphere. Discovered by Heinz Hopf in 1931, it is an influential early example of a fiber bundle. Technically, Hopf found a many-to-one continuous function (or "map") from the 3-sphere onto the 2-sphere such that each distinct point of the 2-sphere is mapped from a distinct great circle of the 3-sphere. Thus the 3-sphere is composed of fibers, where each fiber is a circle — one for each point of the 2-sphere. This fiber bundle structure is denoted meaning that the fiber space S1 (a circle) is embedded in the total space S3 (the 3-sphere), and p : S3 → S2 (Hopf's map) projects S3 onto the base space S2 (the ordinary 2-sphere). The Hopf fibration, like any fiber bundle, has the important property that it is locally a product space. However it is not a trivial fiber bundle, i.e., S3 is not globally a product of S2 and S1 although locally it is indistinguishable from it. This has many implications: for example the existence of this bundle shows that the higher homotopy groups of spheres are not trivial in general. It also provides a basic example of a principal bundle, by identifying the fiber with the circle group. Stereographic projection of the Hopf fibration induces a remarkable structure on R3, in which all of 3-dimensional space, except for the z-axis, is filled with nested tori made of linking Villarceau circles. Here each fiber projects to a circle in space (one of which is a line, thought of as a "circle through infinity"). Each torus is the stereographic projection of the inverse image of a circle of latitude of the 2-sphere. (Topologically, a torus is the product of two circles.) These tori are illustrated in the images at right. When R3 is compressed to the boundary of a ball, some geometric structure is lost although the topological structure is retained (see Topology and geometry). The loops are homeomorphic to circles, although they are not geometric circles. There are numerous generalizations of the Hopf fibration. The unit sphere in complex coordinate space Cn+1 fibers naturally over the complex projective space CPn with circles as fibers, and there are also real, quaternionic, and octonionic versions of these fibrations. In particular, the Hopf fibration belongs to a family of four fiber bundles in which the total space, base space, and fiber space are all spheres: By Adams's theorem such fibrations can occur only in these dimensions. The Hopf fibration is important in twistor theory. (Wikipedia).