
This is a video I have been wanting to make for some time, in which I discuss what the quaternions are, as mathematical objects, and how we do calculations with them. In particular, we will see how the fundamental equation of the quaternions i^2=j^2=k^2=ijk=-1 easily generates the rule for
From playlist Quaternions

Set Theory (Part 14b): Quaternions and 3D Rotations
No background in sets needed for this video - learn about the foundations of quaternions, derivation of the Hamilton product, and their application to 3D rotations. We will also see how dot and cross products are related to quaternion math. This video will be of particular interest to comp
From playlist Set Theory by Mathoma
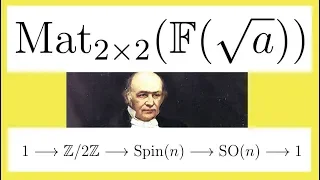
Quaternion algebras via their Mat2x2(F) representations
In this video we talk about general quaternion algebras over a field, their most important properties and how to think about them. The exponential map into unitary groups are covered. I emphasize the Hamiltionion quaternions and motivate their relation to the complex numbers. I conclude wi
From playlist Algebra

The rotation problem and Hamilton's discovery of quaternions III | Famous Math Problems 13c
This is the third lecture on the problem of how to extend the algebraic structure of the complex numbers to deal with rotations in space, and Hamilton's discovery of quaternions, and here we roll up the sleaves and get to work laying out a concise but logically clear framework for this rem
From playlist Famous Math Problems
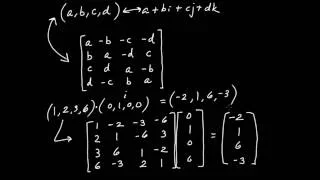
Quaternions as 4x4 Matrices - Connections to Linear Algebra
In math, it's usually possible to view an object or concept from many different (but equivalent) angles. In this video, we will see that the quaternions may be viewed as 4x4 real-valued matrices of a special form. What is interesting here is that if you know how to multiply matrices, you a
From playlist Quaternions

The rotation problem and Hamilton's discovery of quaternions I | Famous Math Problems 13a
W. R. Hamilton in 1846 famously carved the basic multiplicative laws of the four dimensional algebra of quaternions onto a bridge in Dublin during a walk with his wife. This represented a great breakthrough on an important problem he had been wrestling with: how to algebraically represent
From playlist Famous Math Problems

The rotation problem and Hamilton's discovery of quaternions (II) | Famous Math Problems 13b
This is the second of three lectures on Hamilton's discovery of quaternions, and here we introduce rotations of three dimensional space and the natural problem of how to describe them effectively and compose them. We discuss the geometry of the sphere, take a detour to talk about composing
From playlist Famous Math Problems

The rotation problem and Hamilton's discovery of quaternions IV | Famous Math Problems 13d
We show how to practically implement the use of quaternions to describe the algebra of rotations of three dimensional space. The key idea is to use the notion of half-turn [or half-slope--I have changed terminology since this video was made!] instead of angle: this is well suited to connec
From playlist Famous Math Problems

GSE statistics without spin - Sebastian Mueller
Sebastian Mueller University of Bristol November 5, 2013 For more videos, please visit http://video.ias.edu
From playlist Mathematics

Real and symmetric Springer theory - David Nadler
Virtual Workshop on Recent Developments in Geometric Representation Theory Topic: Real and symmetric Springer theory Speaker: David Nadler Affiliation: University of California, Berkeley Date: November 20, 2020 For more video please visit http://video.ias.edu
From playlist Virtual Workshop on Recent Developments in Geometric Representation Theory

Moving on from Lagrange's equation, I show you how to derive Hamilton's equation.
From playlist Physics ONE

Gergely Harcos - A glimpse at arithmetic quantum chaos
slides for this talk: https://www.msri.org/workshops/801/schedules/21771/documents/2987/assets/27969 Introductory Workshop: Analytic Number Theory February 06, 2017 - February 10, 2017 February 07, 2017 (11:00 AM PST - 12:00 PM PST) Speaker(s): Gergely Harcos (Central European Universit
From playlist Number Theory

Caustics of Lagrangian homotopy spheres with stably trivial Gauss map - Daniel Alvarez-Gavela
Joint IAS/Princeton/Montreal/Paris/Tel-Aviv Symplectic Geometry Topic: Caustics of Lagrangian homotopy spheres with stably trivial Gauss map Speaker: Daniel Alvarez-Gavela Date: May 14, 2021 For more video please visit https://www.ias.edu/video
From playlist Mathematics

Random Matrix Theory and its Applications by Satya Majumdar ( Lecture 3 )
PROGRAM BANGALORE SCHOOL ON STATISTICAL PHYSICS - X ORGANIZERS : Abhishek Dhar and Sanjib Sabhapandit DATE : 17 June 2019 to 28 June 2019 VENUE : Ramanujan Lecture Hall, ICTS Bangalore This advanced level school is the tenth in the series. This is a pedagogical school, aimed at bridgin
From playlist Bangalore School on Statistical Physics - X (2019)
Line operators and geometry in 3d N=4 gauge theory by Tudor Dimofte
Program: Quantum Fields, Geometry and Representation Theory ORGANIZERS : Aswin Balasubramanian, Saurav Bhaumik, Indranil Biswas, Abhijit Gadde, Rajesh Gopakumar and Mahan Mj DATE & TIME : 16 July 2018 to 27 July 2018 VENUE : Madhava Lecture Hall, ICTS, Bangalore The power of symmetries
From playlist Quantum Fields, Geometry and Representation Theory

Holomorphic Floer theory and the Fueter equation - Aleksander Doan
Joint IAS/Princeton University Symplectic Geometry Seminar Holomorphic Floer theory and the Fueter equation Aleksander Doan Columbia University Date: April 25, 2022 I will discuss an idea of constructing a category associated with a pair of holomorphic Lagrangians in a hyperkahler manif
From playlist Mathematics

A hitchin-kobayashi correspondance for generalized seiberg-witten equations by Varun Thakre
Program : Integrable systems in Mathematics, Condensed Matter and Statistical Physics ORGANIZERS : Alexander Abanov, Rukmini Dey, Fabian Essler, Manas Kulkarni, Joel Moore, Vishal Vasan and Paul Wiegmann DATE & TIME : 16 July 2018 to 10 August 2018 VENUE : Ramanujan L
From playlist Integrable systems in Mathematics, Condensed Matter and Statistical Physics

Derivation of Hamilton's Equations of Motion | Classical Mechanics
Hamilton’s equations of motion describe how a physical system will evolve over time if you know about the Hamiltonian of this system. 00:00 Introduction 00:12 Prerequisites 01:01 Derivation 01:47 Comparing Coefficients 02:27 Example If you want to read more about the Lagrangian form
From playlist Classical Mechanics

This is lecture 9 of an online mathematics course on groups theory. It covers the quaternions group and its realtion to the ring of quaternions.
From playlist Group theory
