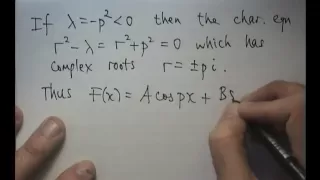Parabolic partial differential equations
Heat equation
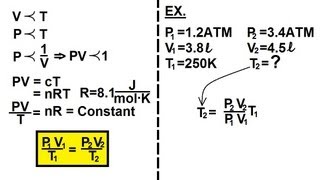
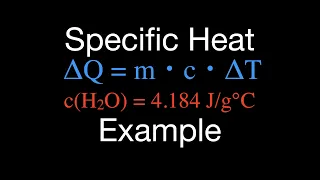
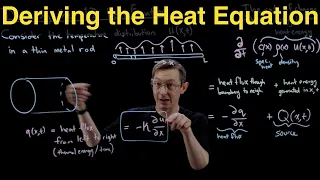
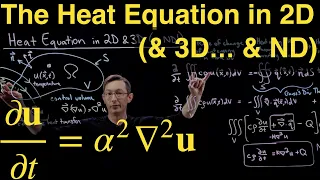
In mathematics and physics, the heat equation is a certain partial differential equation. Solutions of the heat equation are sometimes known as caloric functions. The theory of the heat equation was first developed by Joseph Fourier in 1822 for the purpose of modeling how a quantity such as heat diffuses through a given region. As the prototypical parabolic partial differential equation, the heat equation is among the most widely studied topics in pure mathematics, and its analysis is regarded as fundamental to the broader field of partial differential equations. The heat equation can also be considered on Riemannian manifolds, leading to many geometric applications. Following work of Subbaramiah Minakshisundaram and Åke Pleijel, the heat equation is closely related with spectral geometry. A seminal nonlinear variant of the heat equation was introduced to differential geometry by James Eells and Joseph Sampson in 1964, inspiring the introduction of the Ricci flow by Richard Hamilton in 1982 and culminating in the proof of the Poincaré conjecture by Grigori Perelman in 2003. Certain solutions of the heat equation known as heat kernels provide subtle information about the region on which they are defined, as exemplified through their application to the Atiyah–Singer index theorem. The heat equation, along with variants thereof, is also important in many fields of science and applied mathematics. In probability theory, the heat equation is connected with the study of random walks and Brownian motion via the Fokker–Planck equation. The Black–Scholes equation of financial mathematics is a small variant of the heat equation, and the Schrödinger equation of quantum mechanics can be regarded as a heat equation in imaginary time. In image analysis, the heat equation is sometimes used to resolve pixelation and to identify edges. Following Robert Richtmyer and John von Neumann's introduction of "artificial viscosity" methods, solutions of heat equations have been useful in the mathematical formulation of hydrodynamical shocks. Solutions of the heat equation have also been given much attention in the numerical analysis literature, beginning in the 1950s with work of Jim Douglas, D.W. Peaceman, and Henry Rachford Jr. (Wikipedia).