
Math 101 Fall 2017 112917 Introduction to Compact Sets
Definition of an open cover. Definition of a compact set (in the real numbers). Examples and non-examples. Properties of compact sets: compact sets are bounded. Compact sets are closed. Closed subsets of compact sets are compact. Infinite subsets of compact sets have accumulation poi
From playlist Course 6: Introduction to Analysis (Fall 2017)

Every Compact Set in n space is Bounded
Every Compact Set in n space is Bounded If you enjoyed this video please consider liking, sharing, and subscribing. You can also help support my channel by becoming a member https://www.youtube.com/channel/UCr7lmzIk63PZnBw3bezl-Mg/join Thank you:)
From playlist Advanced Calculus

Introduction to Sets and Set Notation
This video defines a set, special sets, and set notation.
From playlist Sets (Discrete Math)

Introduction to sets || Set theory Overview - Part 1
A set is the mathematical model for a collection of different things; a set contains elements or members, which can be mathematical objects of any kind: numbers, symbols, points in space, lines, other geometrical shapes, variables, or even other #sets. The #set with no element is the empty
From playlist Set Theory

Math 101 Introduction to Analysis 112515: Introduction to Compact Sets
Introduction to Compact Sets: open covers; examples of finite and infinite open covers; definition of compactness; example of a non-compact set; compact implies closed; closed subset of compact set is compact; continuous image of a compact set is compact
From playlist Course 6: Introduction to Analysis

Finding Closed Sets, the Closure of a Set, and Dense Subsets Topology
Please Subscribe here, thank you!!! https://goo.gl/JQ8Nys Finding Closed Sets, the Closure of a Set, and Dense Subsets Topology
From playlist Topology

Introduction to sets || Set theory Overview - Part 2
A set is the mathematical model for a collection of different things; a set contains elements or members, which can be mathematical objects of any kind: numbers, symbols, points in space, lines, other geometrical shapes, variables, or even other #sets. The #set with no element is the empty
From playlist Set Theory

Every Set is an Element of its Power Set | Set Theory
Every set is an element of its own power set. This is because the power set of a set S, P(S), contains all subsets of S. By definition, every set is a subset of itself, and thus by definition of the power set of S, it must contain S. This is even true for the always-fun empty set! We discu
From playlist Set Theory
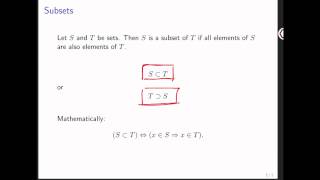
9.3.1 Sets: Definitions and Notation
9.3.1 Sets: Definitions and Notation
From playlist LAFF - Week 9

The Green-Tao theorem and a relative Szemeredi theorem - Yufei Zhao
Slides for this talk: https://drive.google.com/file/d/1RdgY6N869MN5lJwl2jv1HwIgWky6aW5C/view?usp=sharing The Green-Tao theorem and a relative Szemeredi theorem - Yufei Zhao Abstract: The celebrated Green-Tao theorem states that there are arbitrarily long arithmetic progressions in the p
From playlist Mathematics

The Green-Tao theorem and a relative Szemeredi theorem - Yufei Zhao
Yufei Zhao Massachusetts Institute of Technology March 3, 2014 The celebrated Green-Tao theorem states that there are arbitrarily long arithmetic progressions in the primes. In this talk, I will explain the ideas of the proof and discuss our recent simplifications. One of the main ingredie
From playlist Mathematics

Recursively Applying Constructive Dense Model Theorems and Weak Regularity - Russell Impagliazzo
Russell Impagliazzo University of California, San Diego; Member, School of Mathematics February 7, 2011 For more videos, visit http://video.ias.edu
From playlist Mathematics

Learning models: connections between boosting...and regularity II - Russell Impagliazzo
Computer Science/Discrete Mathematics Seminar II Topic: Learning models: connections between boosting...regularity II Speaker: Russell Impagliazzo Affiliation: University of California, San Diego Date: November 14, 2017 For more videos, please visit http://video.ias.edu
From playlist Mathematics

Online List Labeling: Breaking the log2 n Barrier - Nicole Wein
Computer Science/Discrete Mathematics Seminar II Topic: Online List Labeling: Breaking the log2 n Barrier Speaker: Nicole Wein Affiliation: Rutgers University Date: December 06, 2022 The online list labeling problem is a basic primitive in data structures. The goal is to store a dynami
From playlist Mathematics

Medical Search Engine with SPLADE + Sentence Transformers in Python
In this video we'll build a search engine for the medical field using hybrid search with NLP information retrieval models. We use hybrid search with sentence transformers and SPLADE for medical quesiton-answering. By using hybrid search we're able to search using both dense and sparse vec
From playlist ML Projects

Matthew Foreman: Welch games to Laver ideals
Recorded during the meeting "XVI International Luminy Workshop in Set Theory" the September 16, 2021 by the Centre International de Rencontres Mathématiques (Marseille, France) Filmmaker: Guillaume Hennenfent Find this video and other talks given by worldwide mathematicians on CIRM's Au
From playlist Logic and Foundations

The perfect number of axioms | Axiomatic Set Theory, Section 1.1
In this video we introduce 6 of the axioms of ZFC set theory. My Twitter: https://twitter.com/KristapsBalodi3 Intro: (0:00) The Axiom of Existence: (2:39) The Axiom of Extensionality: (4:20) The Axiom Schema of Comprehension: (6:15) The Axiom of Pair (12:16) The Axiom of Union (15:15) T
From playlist Axiomatic Set Theory

Deep Learning for Natural Language Processing with Jon Krohn
Jon Krohn introduces how to preprocess natural language data. He then uses hands-on code demos to build deep learning networks that make predictions using those data. This lesson is an excerpt from "Deep Learning for Natural Language Processing LiveLessons, 2nd Edition." Purchase entire
From playlist Talks and Tutorials
