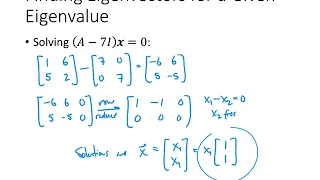
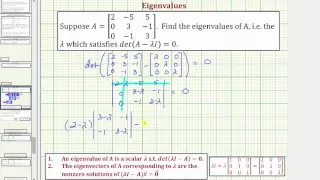
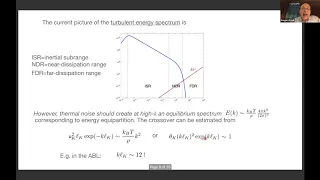
Statistical mechanics theorems
Equipartition theorem
In classical statistical mechanics, the equipartition theorem relates the temperature of a system to its average energies. The equipartition theorem is also known as the law of equipartition, equipartition of energy, or simply equipartition. The original idea of equipartition was that, in thermal equilibrium, energy is shared equally among all of its various forms; for example, the average kinetic energy per degree of freedom in translational motion of a molecule should equal that in rotational motion. The equipartition theorem makes quantitative predictions. Like the virial theorem, it gives the total average kinetic and potential energies for a system at a given temperature, from which the system's heat capacity can be computed. However, equipartition also gives the average values of individual components of the energy, such as the kinetic energy of a particular particle or the potential energy of a single spring. For example, it predicts that every atom in a monatomic ideal gas has an average kinetic energy of 3/2kBT in thermal equilibrium, where kB is the Boltzmann constant and T is the (thermodynamic) temperature. More generally, equipartition can be applied to any classical system in thermal equilibrium, no matter how complicated. It can be used to derive the ideal gas law, and the Dulong–Petit law for the specific heat capacities of solids. The equipartition theorem can also be used to predict the properties of stars, even white dwarfs and neutron stars, since it holds even when relativistic effects are considered. Although the equipartition theorem makes accurate predictions in certain conditions, it is inaccurate when quantum effects are significant, such as at low temperatures. When the thermal energy kBT is smaller than the quantum energy spacing in a particular degree of freedom, the average energy and heat capacity of this degree of freedom are less than the values predicted by equipartition. Such a degree of freedom is said to be "frozen out" when the thermal energy is much smaller than this spacing. For example, the heat capacity of a solid decreases at low temperatures as various types of motion become frozen out, rather than remaining constant as predicted by equipartition. Such decreases in heat capacity were among the first signs to physicists of the 19th century that classical physics was incorrect and that a new, more subtle, scientific model was required. Along with other evidence, equipartition's failure to model black-body radiation—also known as the ultraviolet catastrophe—led Max Planck to suggest that energy in the oscillators in an object, which emit light, were quantized, a revolutionary hypothesis that spurred the development of quantum mechanics and quantum field theory. (Wikipedia).