Real analysis | Infinity | Topological spaces | Projective geometry
Projectively extended real line
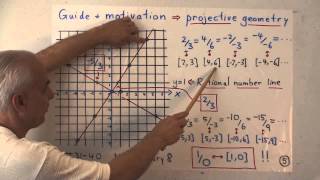
In real analysis, the projectively extended real line (also called the one-point compactification of the real line), is the extension of the set of the real numbers, by a point denoted ∞. It is thus the set with the standard arithmetic operations extended where possible, and is sometimes denoted by The added point is called the point at infinity, because it is considered as a neighbour of both ends of the real line. More precisely, the point at infinity is the limit of every sequence of real numbers whose absolute values are increasing and unbounded. The projectively extended real line may be identified with a real projective line in which three points have been assigned the specific values 0, 1 and ∞. The projectively extended real number line is distinct from the affinely extended real number line, in which +∞ and −∞ are distinct. (Wikipedia).