
Eyal Markman: Hyperholomorphic sheaves and generalized deformations of K3 surfaces
This talk will elaborate on the role hyperholomorphic sheaves play in generalized deformations of K3 surfaces, described in the talk of Sukhendu Mehrotra. The lecture was held within the framework of the Junior Hausdorff Trimester Program Algebraic Geometry. (12.2.2014)
From playlist HIM Lectures: Junior Trimester Program "Algebraic Geometry"

Yinhuo Zhang: Braided autoequivalences, quantum commutative Galois objects and the Brauer groups
Find this video and other talks given by worldwide mathematicians on CIRM's Audiovisual Mathematics Library: http://library.cirm-math.fr. And discover all its functionalities: - Chapter markers and keywords to watch the parts of your choice in the video - Videos enriched with abstracts, b
From playlist Algebra

Abstract Algebra: The definition of a Ring
Learn the definition of a ring, one of the central objects in abstract algebra. We give several examples to illustrate this concept including matrices and polynomials. Be sure to subscribe so you don't miss new lessons from Socratica: http://bit.ly/1ixuu9W ♦♦♦♦♦♦♦♦♦♦ We recommend th
From playlist Abstract Algebra

Pavel Safronov: Quantum character varieties at roots of unity
Abstract: Character varieties of closed surfaces have a natural Poisson structure whose quantization may be constructed in terms of the corresponding quantum group. When the quantum parameter is a root of unity, this quantization carries a central subalgebra isomorphic to the algebra of fu
From playlist Algebraic and Complex Geometry

Group Definition (expanded) - Abstract Algebra
The group is the most fundamental object you will study in abstract algebra. Groups generalize a wide variety of mathematical sets: the integers, symmetries of shapes, modular arithmetic, NxM matrices, and much more. After learning about groups in detail, you will then be ready to contin
From playlist Abstract Algebra

Brauer groups, Severi-Brauer schemes, Azumaya algebras, twisted sheaves
From playlist Étale cohomology and the Weil conjectures

Sachio Ohkawa - On log Cartier transform of higher level in positive characteristic
In this talk, we construct a splitting module of the Azumaya algebra of log differential operators of higher level in characteristic 𝑝 greater than or equal 0 under the assumption of an existence of certain mod 𝑝2 lifting. As an application, we construct a Simpson type correspondence in c
From playlist Journées de géométrie arithmétique de l'IHÉS
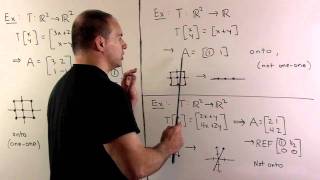
Linear Algebra: Continuing with function properties of linear transformations, we recall the definition of an onto function and give a rule for onto linear transformations.
From playlist MathDoctorBob: Linear Algebra I: From Linear Equations to Eigenspaces | CosmoLearning.org Mathematics

Marcello Bernardara: Semiorthogonal decompositions and birational geometry of geometrically rational
Abstract:This is a joint work in progress with A. Auel. Let S be a geometrically rational del Pezzo surface over a field k. In this talk, I will show how the k-rationality of S is equivalent to the existence of some semiorthogonal decompositions of its derived category. In particular, the
From playlist HIM Lectures: Junior Trimester Program "Algebraic Geometry"

What is a Module? (Abstract Algebra)
A module is a generalization of a vector space. You can think of it as a group of vectors with scalars from a ring instead of a field. In this lesson, we introduce the module, give a variety of examples, and talk about the ways in which modules and vector spaces are different from one an
From playlist Abstract Algebra

Brauer groups, Tsen's theorem, Tate's theorem, finished computing étale cohomology of curves
From playlist Étale cohomology and the Weil conjectures

Ring Definition (expanded) - Abstract Algebra
A ring is a commutative group under addition that has a second operation: multiplication. These generalize a wide variety of mathematical objects like the integers, polynomials, matrices, modular arithmetic, and more. In this video we will take an in depth look at the definition of a rin
From playlist Abstract Algebra

Algebra Absolute Value Equations b6p2
Algebra Absolute Value Equations b6p2 GoToMath.com
From playlist Algebra Gotomath

Field Definition (expanded) - Abstract Algebra
The field is one of the key objects you will learn about in abstract algebra. Fields generalize the real numbers and complex numbers. They are sets with two operations that come with all the features you could wish for: commutativity, inverses, identities, associativity, and more. They
From playlist Abstract Algebra

What is Abstract Algebra? (Modern Algebra)
Abstract Algebra is very different than the algebra most people study in high school. This math subject focuses on abstract structures with names like groups, rings, fields and modules. These structures have applications in many areas of mathematics, and are being used more and more in t
From playlist Abstract Algebra

Units in a Ring (Abstract Algebra)
The units in a ring are those elements which have an inverse under multiplication. They form a group, and this “group of units” is very important in algebraic number theory. Using units you can also define the idea of an “associate” which lets you generalize the fundamental theorem of ar
From playlist Abstract Algebra

8ECM Invited Lecture: Stuart White
From playlist 8ECM Invited Lectures

FIT2.3.3. Algebraic Extensions
Field Theory: We define an algebraic extension of a field F and show that successive algebraic extensions are also algebraic. This gives a useful criterion for checking algberaic elements. We finish with algebraic closures.
From playlist Abstract Algebra

Dihedral Group (Abstract Algebra)
The Dihedral Group is a classic finite group from abstract algebra. It is a non abelian groups (non commutative), and it is the group of symmetries of a regular polygon. This group is easy to work with computationally, and provides a great example of one connection between groups and geo
From playlist Abstract Algebra

Kristin Courtney: "The abstract approach to classifying C*-algebras"
Actions of Tensor Categories on C*-algebras 2021 Mini Course: "The abstract approach to classifying C*-algebras" Kristin Courtney - Westfälische Wilhelms-Universität Münster Institute for Pure and Applied Mathematics, UCLA January 21, 2021 For more information: https://www.ipam.ucla.edu
From playlist Actions of Tensor Categories on C*-algebras 2021