
This lecture is part of an online algebraic geometry course on schemes, based on chapter II of "Algebraic geometry" by Hartshorne. We define the cotangent sheaf of a scheme, and calculate it for the projective line and then for general projective space.
From playlist Algebraic geometry II: Schemes

Graphing the Cotangent Function
Illustrations the graph of the cotangent function using the cotangent segment. Explains how to graph cotangent using reciprocal values of the tangent function http://mathispower4u.wordpress.com/
From playlist Graphing Trigonometric Functions

The TRUTH about TENSORS, Part 9: Vector Bundles
In this video we define vector bundles in full abstraction, of which tangent bundles are a special case.
From playlist The TRUTH about TENSORS
Monotone Lagrangians in cotangent bundles - Luis Diogo
Princeton/IAS Symplectic Geometry Seminar Speaker: Luis Diogo Affiliation: Columbia University Topic: Monotone Lagrangians in cotangent bundles Date: Oct 11, 2016 Description:* We show that there is a 1-parameter family of monotone Lagrangian tori in the cotangent bundle of the 3-sphere w
From playlist Mathematics
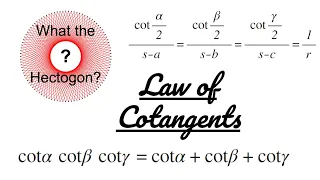
Trigonometry X: the Law of Cotangents (and another lovely relation involving cotangents!)
Boy, oh boy this is a longish one. I prove the Law of Cotangents using the incenter of the triangle, after motivating the path with another way one might seek to prove the Law of Sines using the circumcenter of the triangle. After that, I demonstrate an equally lovely relationship betwee
From playlist Trigonometry
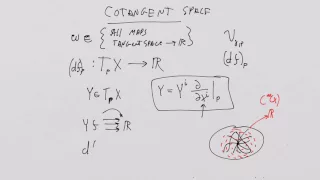
What is a Manifold? Lesson 11: The Cotangent Space
What is a Manifold? Lesson 11: The Cotangent Space I have annotated an obvious error at 2:00. However annotations are not visible on mobile devices!
From playlist What is a Manifold?

Some of the links below are affiliate links. As an Amazon Associate I earn from qualifying purchases. If you purchase through these links, it won't cost you any additional cash, but it will help to support my channel. Thank you! ►PRODUCT RECOMMENDATIONS https://www.amazon.com/shop/brithem
From playlist Calc 1

What is a Manifold? Lesson 12: Fiber Bundles - Formal Description
This is a long lesson, but it is not full of rigorous proofs, it is just a formal definition. Please let me know where the exposition is unclear. I din't quite get through the idea of the structure group of a fiber bundle fully, but I introduced it. The examples in the next lesson will h
From playlist What is a Manifold?

Trigonometry 8 The Tangent and Cotangent of the Sum and Difference of Two Angles.mov
Derive the tangent and cotangent trigonometric identities.
From playlist Trigonometry

Winter School JTP: Introduction to Fukaya categories, James Pascaleff, Lecture 1
This minicourse will provide an introduction to Fukaya categories. I will assume that participants are also attending Keller’s course on A∞ categories. Lecture 1: Basics of symplectic geometry for Fukaya categories. Symplectic manifolds; Lagrangian submanifolds; exactness conditions;
From playlist Winter School on “Connections between representation Winter School on “Connections between representation theory and geometry"

Rahul Pandharipande - Enumerative Geometry of Curves, Maps, and Sheaves 1/5
The main topics will be the intersection theory of tautological classes on moduli space of curves, the enumeration of stable maps via Gromov-Witten theory, and the enumeration of sheaves via Donaldson-Thomas theory. I will cover a mix of classical and modern results. My goal will be, by th
From playlist 2021 IHES Summer School - Enumerative Geometry, Physics and Representation Theory

Index Theory, survey - Stephan Stolz [2018]
TaG survey series These are short series of lectures focusing on a topic in geometry and topology. May_8_2018 Stephan Stolz - Index Theory https://www3.nd.edu/~math/rtg/tag.html (audio fixed)
From playlist Mathematics

Geordie Williamson: Langlands and Bezrukavnikov II Lecture 11
SMRI Seminar Series: 'Langlands correspondence and Bezrukavnikov’s equivalence' Geordie Williamson (University of Sydney) Abstract: The second part of the course focuses on affine Hecke algebras and their categorifications. Last year I discussed the local Langlands correspondence in bro
From playlist Geordie Williamson: Langlands correspondence and Bezrukavnikov’s equivalence

Generation criteria for the Fukaya category II - Mohammed Abouzaid
Mohammed Abouzaid MIT May 12, 2011 For more videos, visit http://video.ias.edu
From playlist Mathematics

Constructions in symplectic and contact topology via h-principles - Oleg Lazarev
More videos on http://video.ias.edu
From playlist Mathematics

Ana Balibanu: The partial compactification of the universal centralizer
Abstract: Let G be a semisimple algebraic group of adjoint type. The universal centralizer is the family of centralizers in G of regular elements in Lie(G), parametrized by their conjugacy classes. It has a natural symplectic structure, obtained by Hamiltonian reduction from the cotangent
From playlist Algebra

Will Sawin - Bounding the stalks of perverse sheaves in characteristic p via the (...)
The sheaf-function dictionary shows that many natural functions on the F_q-points of a variety over F_q can be obtained from l-adic sheaves on that variety. To obtain upper bounds on these functions, it is necessary to obtain upper bounds on the dimension of the stalks of these sheaves. In
From playlist Franco-Asian Summer School on Arithmetic Geometry (CIRM)

Integral of cot^5x, cot(x) and csc(x) approach
integral of cot^5(x), integral of (cot(x))^5, integral of cotangent, playlist: https://www.youtube.com/playlist?list=PLj7p5OoL6vGzK7feKUEtd_BUmTCCf5tlo blackpenredpen
From playlist Calculus: Sect 7.2 Trigonometric Integrals, Stewart Calculus Solution, 7th ET edition