Sullivan conjecture

Fixed points (mathematics) | Conjectures that have been proved | Homotopy theory

In mathematics, Sullivan conjecture or Sullivan's conjecture on maps from classifying spaces can refer to any of several results and conjectures prompted by homotopy theory work of Dennis Sullivan. A basic theme and motivation concerns the fixed point set in group actions of a finite group . The most elementary formulation, however, is in terms of the classifying space of such a group. Roughly speaking, it is difficult to map such a space continuously into a finite CW complex in a non-trivial manner. Such a version of the Sullivan conjecture was first proved by Haynes Miller. Specifically, in 1984, Miller proved that the function space, carrying the compact-open topology, of base point-preserving mappings from to is weakly contractible. This is equivalent to the statement that the map → from X to the function space of maps → , not necessarily preserving the base point, given by sending a point of to the constant map whose image is is a weak equivalence. The mapping space is an example of a homotopy fixed point set. Specifically, is the homotopy fixed point set of the group acting by the trivial action on . In general, for a group acting on a space , the homotopy fixed points are the fixed points of the mapping space of maps from the universal cover of to under the -action on given by in acts on a map in by sending it to . The -equivariant map from to a single point induces a natural map η: → from the fixed points to the homotopy fixed points of acting on . Miller's theorem is that η is a weak equivalence for trivial -actions on finite-dimensional CW complexes. An important ingredient and motivation for his proof is a result of Gunnar Carlsson on the homology of as an unstable module over the Steenrod algebra. Miller's theorem generalizes to a version of Sullivan's conjecture in which the action on is allowed to be non-trivial. In, Sullivan conjectured that η is a weak equivalence after a certain p-completion procedure due to A. Bousfield and D. Kan for the group . This conjecture was incorrect as stated, but a correct version was given by Miller, and proven independently by Dwyer-Miller-Neisendorfer, Carlsson, and Jean Lannes, showing that the natural map → is a weak equivalence when the order of is a power of a prime p, and where denotes the Bousfield-Kan p-completion of . Miller's proof involves an unstable Adams spectral sequence, Carlsson's proof uses his affirmative solution of the Segal conjecture and also provides information about the homotopy fixed points before completion, and Lannes's proof involves his T-functor. (Wikipedia).

What is the Riemann Hypothesis?

This video provides a basic introduction to the Riemann Hypothesis based on the the superb book 'Prime Obsession' by John Derbyshire. Along the way I look at convergent and divergent series, Euler's famous solution to the Basel problem, and the Riemann-Zeta function. Analytic continuation

From playlist Mathematics

A (compelling?) reason for the Riemann Hypothesis to be true #SOME2

A visual walkthrough of the Riemann Zeta function and a claim of a good reason for the truth of the Riemann Hypothesis. This is not a formal proof but I believe the line of argument could lead to a formal proof.

From playlist Summer of Math Exposition 2 videos

(ML 19.2) Existence of Gaussian processes

Statement of the theorem on existence of Gaussian processes, and an explanation of what it is saying.

From playlist Machine Learning

The Most Difficult Math Problem You've Never Heard Of - Birch and Swinnerton-Dyer Conjecture

The Birch and Swinnerton-Dyer Conjecture is a millennium prize problem, one of the famed seven placed by the Clay Mathematical Institute in the year 2000. As the only number-theoretic problem in the list apart from the Riemann Hypothesis, the BSD Conjecture has been haunting mathematicians

From playlist Math

Rigidity of the hexagonal triangulation of the plane and its applications - Feng Luo

Feng Luo, Rutgers October 5, 2015 http://www.math.ias.edu/wgso3m/agenda 015-2016 Monday, October 5, 2015 - 08:00 to Friday, October 9, 2015 - 12:00 This workshop is part of the topical program "Geometric Structures on 3-Manifolds" which will take place during the 2015-2016 academic year

From playlist Workshop on Geometric Structures on 3-Manifolds

2022's Biggest Breakthroughs in Math

Mathematicians made major progress in 2022, solving a centuries-old geometry question called the interpolation problem, proving the best way to minimize the surface area of clusters of three, four and five bubbles, and proving a sweeping statement about how structure emerges in random sets

From playlist Discoveries

PIGEONHOLE PRINCIPLE - DISCRETE MATHEMATICS

We introduce the pigeonhole principle, an important proof technique. #DiscreteMath #Mathematics #Proofs #Pigeonhole Visit our website: http://bit.ly/1zBPlvm Subscribe on YouTube: http://bit.ly/1vWiRxW *--Playlists--* Discrete Mathematics 1: https://www.youtube.com/playlist?list=PLDDGPdw

From playlist Discrete Math 1

Interview at CIRM : Curtis McMullen

Interview at CIRM : Curtis McMullen Curtis Tracy McMullen (born 21 May 1958) is Professor of Mathematics at Harvard University. He was awarded the Fields Medal in 1998 for his work in complex dynamics, hyperbolic geometry and Teichmüller theory. McMullen graduated as valedictorian in 1980

From playlist English interviews - Interviews en anglais

Alex Kontorovich - On the Strong Density Conjecture for Apollonian Circle Packings [2012]

slides for this talk: https://docs.google.com/viewer?url=http://www.msri.org/workshops/652/schedules/14560/documents/1681/assets/17223 Abstract: The Strong Density Conjecture states that for a given primitive integral Apollonian circle packing, every sufficiently large admissible (passing

From playlist Number Theory

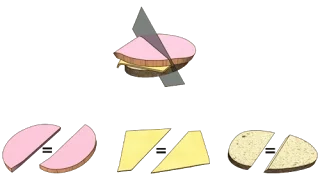
What is the ham sandwich theorem?

From playlist Mathematics

Dennis Sullivan: Gathering chestnuts of math related to fluid motion

This lecture was held by the 2022 Abel Laureate Dennis Sullivan at The University of Oslo, May 25, 2022 and was part of the Abel Prize lectures held in connection with the Abel Prize Week celebrations.

From playlist Dennis Sullivan

Estimating Reeb chords using microlocal sheaf theory - Wenyuan Li

Joint IAS/Princeton/Montreal/Paris/Tel-Aviv Symplectic Geometry Zoominar Topic: Estimating Reeb chords using microlocal sheaf theory SpeakerL: Wenyuan Li Affiliation: Northwestern Date: December 17, 2021 We show that, for closed Legendrians in 1-jet bundles, when there is a sheaf with s

From playlist Mathematics

The Pattern to Prime Numbers?

In this video, we explore the "pattern" to prime numbers. I go over the Euler product formula, the prime number theorem and the connection between the Riemann zeta function and primes. Here's a video on a similar topic by Numberphile if you're interested: https://youtu.be/uvMGZb0Suyc The

From playlist Other Math Videos

Some identities involving the Riemann-Zeta function.

After introducing the Riemann-Zeta function we derive a generating function for its values at positive even integers. This generating function is used to prove two sum identities. http://www.michael-penn.net http://www.randolphcollege.edu/mathematics/

From playlist The Riemann Zeta Function

What is... an elliptic curve?

In this talk, we will define elliptic curves and, more importantly, we will try to motivate why they are central to modern number theory. Elliptic curves are ubiquitous not only in number theory, but also in algebraic geometry, complex analysis, cryptography, physics, and beyond. They were

From playlist An Introduction to the Arithmetic of Elliptic Curves

Gregory Margulis - The Abel Prize interview 2020

00:00 congratulations to Gregory Margulis 01:33 when did you interests in mathematics start? 02:33 growing up in Moscow in the 50’s and 60’s and being included in mathematical circles 05:47 mathematical Olympiads 06:32 early career and the paper with Kazhdan 08:03 Margulis at the Institute

From playlist Gregory Margulis

The 2022 Abel Prize Award Ceremony

The Abel prize award ceremony honours the 2022 Abel prize laureate, Dennis Sullivan. 0:33 Musical performance by String Quartet Saphir 3:30 Welcome by Master of ceremonies, Haddy Njie 04:40 Lise Øvreås, President of The Norwegian Academy of Science and Letters, on the purpose of the Abel

From playlist Dennis Sullivan

Geoffroy Horel - Knots and Motives

The pure braid group is the fundamental group of the space of configurations of points in the complex plane. This topological space is the Betti realization of a scheme defined over the integers. It follows, by work initiated by Deligne and Goncharov, that the pronilpotent completion of th

From playlist Summer School 2020: Motivic, Equivariant and Non-commutative Homotopy Theory

Mikhail Lyubich: Story of the Feigenbaum point

HYBRID EVENT Recorded during the meeting "Advancing Bridges in Complex Dynamics" the September 23, 2021 by the Centre International de Rencontres Mathématiques (Marseille, France) Filmmaker: Luca Récanzone Find this video and other talks given by worldwide mathematicians on CIRM's Audi

From playlist Dynamical Systems and Ordinary Differential Equations

Riemann Sum Defined w/ 2 Limit of Sums Examples Calculus 1

I show how the Definition of Area of a Plane is a special case of the Riemann Sum. When finding the area of a plane bound by a function and an axis on a closed interval, the width of the partitions (probably rectangles) does not have to be equal. I work through two examples that are rela

From playlist Calculus

Sullivan conjecture

Related pages