
Introduction to sets || Set theory Overview - Part 2
A set is the mathematical model for a collection of different things; a set contains elements or members, which can be mathematical objects of any kind: numbers, symbols, points in space, lines, other geometrical shapes, variables, or even other #sets. The #set with no element is the empty
From playlist Set Theory

Fundamentals of Mathematics - Lecture 33: Dedekind's Definition of Infinite Sets are FInite Sets
https://www.uvm.edu/~tdupuy/logic/Math52-Fall2017.html
From playlist Fundamentals of Mathematics

Why is the Empty Set a Subset of Every Set? | Set Theory, Subsets, Subset Definition
The empty set is a very cool and important part of set theory in mathematics. The empty set contains no elements and is denoted { } or with the empty set symbol ∅. As a result of the empty set having no elements is that it is a subset of every set. But why is that? We go over that in this
From playlist Set Theory

Introduction to sets || Set theory Overview - Part 1
A set is the mathematical model for a collection of different things; a set contains elements or members, which can be mathematical objects of any kind: numbers, symbols, points in space, lines, other geometrical shapes, variables, or even other #sets. The #set with no element is the empty
From playlist Set Theory

Set Theory (Part 2b): The Bogus Universal Set
Please feel free to leave comments/questions on the video below! In this video, I argue against the existence of the set of all sets and show that this claim is provable in ZFC. This theorem is very much tied to the Russell Paradox, besides being one of the problematic ideas in mathematic
From playlist Set Theory by Mathoma

Introduction to Sets and Set Notation
This video defines a set, special sets, and set notation.
From playlist Sets (Discrete Math)

Set Theory (Part 20): The Complex Numbers are Uncountably Infinite
Please feel free to leave comments/questions on the video and practice problems below! In this video, we will establish a bijection between the complex numbers and the real numbers, showing that the complex numbers are also uncountably infinite. This will eventually mean that the cardinal
From playlist Set Theory by Mathoma

This lecture is on Introduction to Higher Mathematics (Proofs). For more see http://calculus123.com.
From playlist Proofs
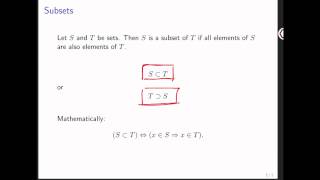
9.3.1 Sets: Definitions and Notation
9.3.1 Sets: Definitions and Notation
From playlist LAFF - Week 9

Lecture 11: The Lebesgue Integral of a Nonnegative Function and Convergence Theorems
MIT 18.102 Introduction to Functional Analysis, Spring 2021 Instructor: Dr. Casey Rodriguez View the complete course: https://ocw.mit.edu/courses/18-102-introduction-to-functional-analysis-spring-2021/ YouTube Playlist: https://www.youtube.com/watch?v=ZWzCHjN3_3s&list=PLUl4u3cNGP63micsJp_
From playlist MIT 18.102 Introduction to Functional Analysis, Spring 2021

Nexus Trimester - Raymond Yeung (The Chinese University of Hong Kong) 1/3
Shannon's Information Measures and Markov Structures Raymond Yeung (The Chinese University of Hong Kong) February 18,2016 Abstract: Most studies of finite Markov random fields assume that the underlying probability mass function (pmf) of the random variables is strictly positive. With thi
From playlist Nexus Trimester - 2016 - Fundamental Inequalities and Lower Bounds Theme

Big fiber theorems and ideal-valued measures in symplectic topology - Yaniv Ganor
Joint IAS/Princeton/Montreal/Paris/Tel-Aviv Symplectic Geometry Zoominar Topic: Big fiber theorems and ideal-valued measures in symplectic topology Speaker: Yaniv Ganor Affiliation: Technion Date: October 22, 2021 In various areas of mathematics there exist "big fiber theorems", these a
From playlist Mathematics

Matthew Kennedy: Noncommutative convexity
Talk by Matthew Kennedy in Global Noncommutative Geometry Seminar (Europe) http://www.noncommutativegeometry.nl/ncgseminar/ on May 5, 2021
From playlist Global Noncommutative Geometry Seminar (Europe)

Lecture 12: Lebesgue Integrable Functions, the Lebesgue Integral and the Dominated Convergence...
MIT 18.102 Introduction to Functional Analysis, Spring 2021 Instructor: Dr. Casey Rodriguez View the complete course: https://ocw.mit.edu/courses/18-102-introduction-to-functional-analysis-spring-2021/ YouTube Playlist: https://www.youtube.com/watch?v=W2pw1JWc9k4&list=PLUl4u3cNGP63micsJp_
From playlist MIT 18.102 Introduction to Functional Analysis, Spring 2021

MIT 18.102 Introduction to Functional Analysis, Spring 2021 Instructor: Dr. Casey Rodriguez View the complete course: https://ocw.mit.edu/courses/18-102-introduction-to-functional-analysis-spring-2021/ YouTube Playlist: https://www.youtube.com/watch?v=TXMCTAF6SEE&list=PLUl4u3cNGP63micsJp_
From playlist MIT 18.102 Introduction to Functional Analysis, Spring 2021

Limiting Distributions of Stretching Translates of Shrinking Curves...by Nimish Shah
PROGRAM : ERGODIC THEORY AND DYNAMICAL SYSTEMS (HYBRID) ORGANIZERS : C. S. Aravinda (TIFR-CAM, Bengaluru), Anish Ghosh (TIFR, Mumbai) and Riddhi Shah (JNU, New Delhi) DATE : 05 December 2022 to 16 December 2022 VENUE : Ramanujan Lecture Hall and Online The programme will have an emphasis
From playlist Ergodic Theory and Dynamical Systems 2022

GPDE Workshop - External doubly stochastic measures and optimal transportation
Robert McCann University of Toronto February 23, 2009 For more videos, visit http://video.ias.edu
From playlist Mathematics

Equidistribution of Measures with High Entropy for General Surface Diffeomorphisms by Omri Sarig
PROGRAM : ERGODIC THEORY AND DYNAMICAL SYSTEMS (HYBRID) ORGANIZERS : C. S. Aravinda (TIFR-CAM, Bengaluru), Anish Ghosh (TIFR, Mumbai) and Riddhi Shah (JNU, New Delhi) DATE : 05 December 2022 to 16 December 2022 VENUE : Ramanujan Lecture Hall and Online The programme will have an emphasis
From playlist Ergodic Theory and Dynamical Systems 2022

MIT 18.102 Introduction to Functional Analysis, Spring 2021 Instructor: Dr. Casey Rodriguez View the complete course: https://ocw.mit.edu/courses/18-102-introduction-to-functional-analysis-spring-2021/ YouTube Playlist: https://www.youtube.com/watch?v=OHiu2F18dFA&list=PLUl4u3cNGP63micsJp_
From playlist MIT 18.102 Introduction to Functional Analysis, Spring 2021
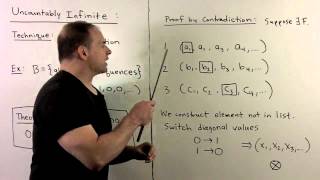
BM9.2. Cardinality 2: Infinite Sets
Basic Methods: We continue the study of cardinality with infinite sets. First the class of countably infinite sets is considered, and basic results given. Then we give examples of uncountable sets using Cantor diagonalization arguments.
From playlist Math Major Basics