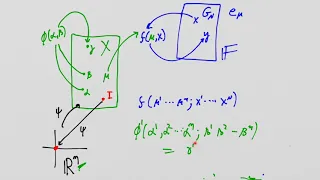
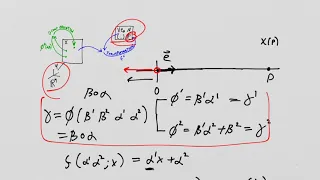
Lie algebra extension
In the theory of Lie groups, Lie algebras and their representation theory, a Lie algebra extension e is an enlargement of a given Lie algebra g by another Lie algebra h. Extensions arise in several ways. There is the trivial extension obtained by taking a direct sum of two Lie algebras. Other types are the split extension and the central extension. Extensions may arise naturally, for instance, when forming a Lie algebra from projective group representations. Such a Lie algebra will contain central charges. Starting with a polynomial loop algebra over finite-dimensional simple Lie algebra and performing two extensions, a central extension and an extension by a derivation, one obtains a Lie algebra which is isomorphic with an untwisted affine Kac–Moody algebra. Using the centrally extended loop algebra one may construct a current algebra in two spacetime dimensions. The Virasoro algebra is the universal central extension of the Witt algebra. Central extensions are needed in physics, because the symmetry group of a quantized system usually is a central extension of the classical symmetry group, and in the same way the corresponding symmetry Lie algebra of the quantum system is, in general, a central extension of the classical symmetry algebra. Kac–Moody algebras have been conjectured to be symmetry groups of a unified superstring theory. The centrally extended Lie algebras play a dominant role in quantum field theory, particularly in conformal field theory, string theory and in M-theory. A large portion towards the end is devoted to background material for applications of Lie algebra extensions, both in mathematics and in physics, in areas where they are actually useful. A parenthetical link,, is provided where it might be beneficial. (Wikipedia).