
Units in a Ring (Abstract Algebra)
The units in a ring are those elements which have an inverse under multiplication. They form a group, and this “group of units” is very important in algebraic number theory. Using units you can also define the idea of an “associate” which lets you generalize the fundamental theorem of ar
From playlist Abstract Algebra

Definition of a Ring and Examples of Rings
Please Subscribe here, thank you!!! https://goo.gl/JQ8Nys Definition of a Ring and Examples of Rings - Definition of a Ring. - Definition of a commutative ring and a ring with identity. - Examples of Rings include: Z, Q, R, C under regular addition and multiplication The Ring of all n x
From playlist Abstract Algebra

Ring Definition (expanded) - Abstract Algebra
A ring is a commutative group under addition that has a second operation: multiplication. These generalize a wide variety of mathematical objects like the integers, polynomials, matrices, modular arithmetic, and more. In this video we will take an in depth look at the definition of a rin
From playlist Abstract Algebra

Abstract Algebra | Types of rings.
We define several and give examples of different types of rings which have additional structure. http://www.michael-penn.net http://www.randolphcollege.edu/mathematics/
From playlist Abstract Algebra

Ring Theory: We define rings and give many examples. Items under consideration include commutativity and multiplicative inverses. Example include modular integers, square matrices, polynomial rings, quaternions, and adjoins of algebraic and transcendental numbers.
From playlist Abstract Algebra

RNT1.4. Ideals and Quotient Rings
Ring Theory: We define ideals in rings as an analogue of normal subgroups in group theory. We give a correspondence between (two-sided) ideals and kernels of homomorphisms using quotient rings. We also state the First Isomorphism Theorem for Rings and give examples.
From playlist Abstract Algebra

Abstract Algebra: The definition of a Ring
Learn the definition of a ring, one of the central objects in abstract algebra. We give several examples to illustrate this concept including matrices and polynomials. Be sure to subscribe so you don't miss new lessons from Socratica: http://bit.ly/1ixuu9W ♦♦♦♦♦♦♦♦♦♦ We recommend th
From playlist Abstract Algebra

Ring Examples (Abstract Algebra)
Rings are one of the key structures in Abstract Algebra. In this video we give lots of examples of rings: infinite rings, finite rings, commutative rings, noncommutative rings and more! Be sure to subscribe so you don't miss new lessons from Socratica: http://bit.ly/1ixuu9W ♦♦♦♦♦♦♦♦♦
From playlist Abstract Algebra

Rings and midules 3: Burnside ring and rings of differential operators
This lecture is part of an online course on rings and modules. We discuss a few assorted examples of rings. The Burnside ring of a group is a ring constructed form the permutation representations. The ring of differentail operators is a ring whose modules are related to differential equat
From playlist Rings and modules
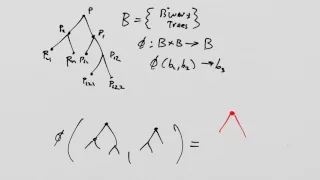
What is a Tensor? Lesson 19: Algebraic Structures I
What is a Tensor? Lesson 19: Algebraic Structures Part One: Groupoids to Fields This is a redo or a recently posted lesson. Same content, a bit cleaner. Algebraic structures are frequently mentioned in the literature of general relativity, so it is good to understand the basic lexicon of
From playlist What is a Tensor?

Rings and modules 4 Unique factorization
This lecture is part of an online course on rings and modules. We discuss unique factorization in rings, showing the implications (Integers) implies (Euclidean domain) implies (Principal ideal domain) implies (Unique factorization domain). We give a few examples to illustrate these implic
From playlist Rings and modules

The importance of Being in Shape - Lecture 2 by Ramanujam Srinivasan
ORGANIZERS : Vidyanand Nanjundiah and Olivier Rivoire DATE & TIME : 16 April 2018 to 26 April 2018 VENUE : Ramanujan Lecture Hall, ICTS Bangalore This program is aimed at Master's- and PhD-level students who wish to be exposed to interesting problems in biology that lie at the biology-
From playlist Living Matter 2018

Nihar Gargava - Random lattices as sphere packings
In 1945, Siegel showed that the expected value of the lattice-sums of a function over all the lattices of unit covolume in an n-dimensional real vector space is equal to the integral of the function. In 2012, Venkatesh restricted the lattice- sum function to a collection of lattices that h
From playlist Combinatorics and Arithmetic for Physics: Special Days 2022

[ANT01] Algebraic number theory: an introduction, via Fermat's last theorem
The existence of the Euclidean algorithm is what makes multiplication in Z so nice. But some other rings have Euclidean algorithms too. Here's how we can exploit this for profit.
From playlist [ANT] An unorthodox introduction to algebraic number theory

Biology and Reconstitution of Minimalistic Systems by R Srinivasan
DISCUSSION MEETING : THIRSTING FOR THEORETICAL BIOLOGY ORGANIZERS : Vijaykumar Krishnamurthy and Vidyanand Nanjundiah DATE : 03 June 2019 to 07 June 2019 VENUE : Ramanujan Lecture Hall, ICTS Bangalore Experimental biologists frequently point out that theoreticians trivialise th
From playlist Thirsting for Theoretical Biology 2019

Rings and modules 2: Group rings
This lecture is part of an online course on rings and modules. We decribe some examples of rings constructed from groups and monoids, such as group rings and rings of Dirichlet polynomials. For the other lectures in the course see https://www.youtube.com/playlist?list=PL8yHsr3EFj52XDLrm
From playlist Rings and modules

Visual Group Theory, Lecture 7.1: Basic ring theory
Visual Group Theory, Lecture 7.1: Basic ring theory A ring is an abelian group (R,+) with a second binary operation, multiplication and the distributive law. Multiplication need not commute, nor need there be multiplicative inverses, so a ring is like a field but without these properties.
From playlist Visual Group Theory

