
Darij Grinberg -Quotients of Symmetric Polynomial Rings Deforming theCohomology of the Grassmannian
One of the many connections between Grassmannians and combinatorics is cohomological: The cohomology ring of a Grassmannian Gr(k,n) is a quotient of the ring S of symmetric polynomials in k variables. More precisely, it is the quotient of S by the ideal generated by the k consecutive compl
From playlist Combinatorics and Arithmetic for Physics: 02-03 December 2020

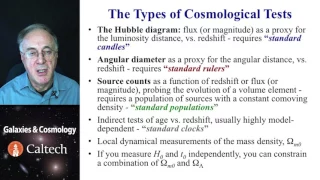
http://www.teachastronomy.com/ Cosmology is the study of the universe, its history, and everything in it. It comes from the Greek root of the word cosmos for order and harmony which reflected the Greek belief that the universe was a harmonious entity where everything worked in concert to
From playlist 22. The Big Bang, Inflation, and General Cosmology

Gysin sequences and cohomology ring of symplectic fillings - Zhengyi Zhou
More videos on http://video.ias.edu
From playlist Mathematics

Ring Theory: We define rings and give many examples. Items under consideration include commutativity and multiplicative inverses. Example include modular integers, square matrices, polynomial rings, quaternions, and adjoins of algebraic and transcendental numbers.
From playlist Abstract Algebra

Definition of a Ring and Examples of Rings
Please Subscribe here, thank you!!! https://goo.gl/JQ8Nys Definition of a Ring and Examples of Rings - Definition of a Ring. - Definition of a commutative ring and a ring with identity. - Examples of Rings include: Z, Q, R, C under regular addition and multiplication The Ring of all n x
From playlist Abstract Algebra

Aurel PAGE - Cohomology of arithmetic groups and number theory: geometric, ... 2
In this lecture series, the first part will be dedicated to cohomology of arithmetic groups of lower ranks (e.g., Bianchi groups), their associated geometric models (mainly from hyperbolic geometry) and connexion to number theory. The second part will deal with higher rank groups, mainly
From playlist École d'Été 2022 - Cohomology Geometry and Explicit Number Theory

Aurel PAGE - Cohomology of arithmetic groups and number theory: geometric, ... 1
In this lecture series, the first part will be dedicated to cohomology of arithmetic groups of lower ranks (e.g., Bianchi groups), their associated geometric models (mainly from hyperbolic geometry) and connexion to number theory. The second part will deal with higher rank groups, mainly
From playlist École d'Été 2022 - Cohomology Geometry and Explicit Number Theory

Paul GUNNELLS - Cohomology of arithmetic groups and number theory: geometric, ... 2
In this lecture series, the first part will be dedicated to cohomology of arithmetic groups of lower ranks (e.g., Bianchi groups), their associated geometric models (mainly from hyperbolic geometry) and connexion to number theory. The second part will deal with higher rank groups, mainly
From playlist École d'Été 2022 - Cohomology Geometry and Explicit Number Theory

Abstract Algebra | The motivation for the definition of an ideal.
Towards the goal of creating a quotient ring, we uncover the defintion of an ideal. http://www.michael-penn.net https://www.researchgate.net/profile/Michael_Penn5 http://www.randolphcollege.edu/mathematics/
From playlist Abstract Algebra
Nero Budur: Cohomology jump loci and singularities
Find this video and other talks given by worldwide mathematicians on CIRM's Audiovisual Mathematics Library: http://library.cirm-math.fr. And discover all its functionalities: - Chapter markers and keywords to watch the parts of your choice in the video - Videos enriched with abstracts, b
From playlist Algebraic and Complex Geometry

Duality for Rabinowitz-Floer homology - Alex Oancea
IAS/PU-Montreal-Paris-Tel-Aviv Symplectic Geometry Topic: Duality for Rabinowitz-Floer homology Speaker: Alex Oancea Affiliation: Institut de Mathématiques de Jussieu-Paris Rive Gauche Date: May 27, 2020 For more video please visit http://video.ias.edu
From playlist PU/IAS Symplectic Geometry Seminar

Jennifer WILSON - High dimensional cohomology of SL_n(Z) and its principal congruence subgroups 1
Group cohomology of arithmetic groups is ubiquitous in the study of arithmetic K-theory and algebraic number theory. Rationally, SL_n(Z) and its finite index subgroups don't have cohomology above dimension n choose 2. Using Borel-Serre duality, one has access to the high dimensions. Church
From playlist École d'Été 2022 - Cohomology Geometry and Explicit Number Theory

B. Bhatt - Prisms and deformations of de Rham cohomology
Prisms are generalizations of perfectoid rings to a setting where "Frobenius need not be an isomorphism". I will explain the definition and use it to construct a prismatic site for any scheme. The resulting prismatic cohomology often gives a one-parameter deformation of de Rham cohomology.
From playlist Arithmetic and Algebraic Geometry: A conference in honor of Ofer Gabber on the occasion of his 60th birthday

Lars Hesselholt: Around topological Hochschild homology (Lecture 8)
The lecture was held within the framework of the (Junior) Hausdorff Trimester Program Topology: "Workshop: Hermitian K-theory and trace methods" Introduced by Bökstedt in the late eighties, topological Hochschild homology is a manifestation of the dual visions of Connes and Waldhausen to
From playlist HIM Lectures: Junior Trimester Program "Topology"

A Gentle Approach to Crystalline Cohomology - Jacob Lurie
Members’ Colloquium Topic: A Gentle Approach to Crystalline Cohomology Speaker: Jacob Lurie Affiliation: Professor, School of Mathematics Date: February 28, 2022 Let X be a smooth affine algebraic variety over the field C of complex numbers (that is, a smooth submanifold of C^n which can
From playlist Mathematics

The cup product operation [Ling Zhou]
In this tutorial, you will learn about the cup product operation in the simplicial setting, and go through an example of computing it in the 2-torus. The cup product induces a ring structure on cohomology, making it more informative than homology. In the TDA community, many attentions have
From playlist Tutorial-a-thon 2021 Fall

Peter PATZT - High dimensional cohomology of SL_n(Z) and its principal congruence subgroups 4
Group cohomology of arithmetic groups is ubiquitous in the study of arithmetic K-theory and algebraic number theory. Rationally, SL_n(Z) and its finite index subgroups don't have cohomology above dimension n choose 2. Using Borel-Serre duality, one has access to the high dimensions. Church
From playlist École d'Été 2022 - Cohomology Geometry and Explicit Number Theory

Lars Hesselholt: Around topological Hochschild homology (Lecture 7)
The lecture was held within the framework of the (Junior) Hausdorff Trimester Program Topology: "Workshop: Hermitian K-theory and trace methods" Introduced by Bökstedt in the late eighties, topological Hochschild homology is a manifestation of the dual visions of Connes and Waldhausen to
From playlist HIM Lectures: Junior Trimester Program "Topology"
From playlist Courses and Series

James Borger: The geometric approach to cohomology Part I
SMRI Seminar Course: 'The geometric approach to cohomology' Part I James Borger (Australian National University) Abstract: The aim of these two talks is to give an overview of the geometric aka stacky approach to various cohomology theories for schemes: de Rham, Hodge, crystalline and pr
From playlist SMRI Course: The geometric approach to cohomology