
Three Partitioning Cases - Intro to Algorithms
This video is part of an online course, Intro to Algorithms. Check out the course here: https://www.udacity.com/course/cs215.
From playlist Introduction to Algorithms
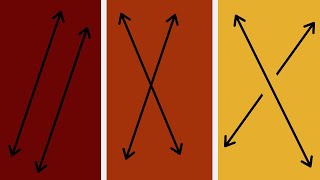
Multivariable Calculus | Interactions of lines in 3 dimensions.
We describe how two lines can interact in three dimensions. In addition, we give examples of intersecting, parallel, and skew lines. http://www.michael-penn.net http://www.randolphcollege.edu/mathematics/
From playlist Lines and Planes in Three Dimensions

A brief introduction to partitions and combinatorics. This video is part of the #MegaFavNumbers project. More videos can be found here: https://www.youtube.com/playlist?list=PLar4u0v66vIodqt3KSZPsYyuULD5meoAo
From playlist MegaFavNumbers

Limits & Continuity (3 of 3: Applications to graphs)
More resources available at www.misterwootube.com
From playlist Introduction to Differentiation

Ratio Division with Vectors (1 of 2: Internal)
More resources available at www.misterwootube.com
From playlist Further Work with Vectors
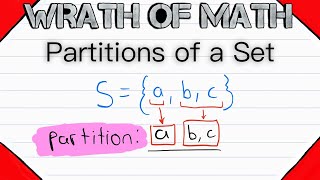
Partitions of a Set | Set Theory
What is a partition of a set? Partitions are very useful in many different areas of mathematics, so it's an important concept to understand. We'll define partitions of sets and give examples in today's lesson! A partition of a set is basically a way of splitting a set completely into disj
From playlist Set Theory

Ex 5: System of Three Equations with Three Unknowns Using Elimination (Infinite Solutions)
This is the first of several examples that will show how to solve a system of three linear equations with three unknowns. The result is shows graphically in 3D. This example has infinite solutions. The solutions are expressed parametrically. Site: http://mathispower4u.com
From playlist Systems of Equations with Three Unknowns

In this video, we take a look at systems with three variables.
From playlist Systems of Equations

Lecture 11 - Stirling & Harmonic Numbers
This is Lecture 11 of the CSE547 (Discrete Mathematics) taught by Professor Steven Skiena [http://www.cs.sunysb.edu/~skiena/] at Stony Brook University in 1999. The lecture slides are available at: http://www.cs.sunysb.edu/~algorith/math-video/slides/Lecture%2011.pdf More information may
From playlist CSE547 - Discrete Mathematics - 1999 SBU

5 Simple Steps for Solving Any Recursive Problem
In this video, we take a look at one of the more challenging computer science concepts: Recursion. We introduce 5 simple steps to help you solve challenging recursive problems and show you 3 specific examples, each progressively more difficult than the last. Support: https://www.patreon.c
From playlist Problem Solving

Introduction to Integer Partitions -- Number Theory 28
Suggest a problem: https://forms.gle/ea7Pw7HcKePGB4my5 Please Subscribe: https://www.youtube.com/michaelpennmath?sub_confirmation=1 Patreon: https://www.patreon.com/michaelpennmath Merch: https://teespring.com/stores/michael-penn-math Personal Website: http://www.michael-penn.net Randolp
From playlist Number Theory v2

Introduction to Integer Partitions -- Number Theory 28
⭐Support the channel⭐ Patreon: https://www.patreon.com/michaelpennmath Merch: https://teespring.com/stores/michael-penn-math My amazon shop: https://www.amazon.com/shop/michaelpenn 🟢 Discord: https://discord.gg/Ta6PTGtKBm ⭐my other channels⭐ Main Channel: https://www.youtube.
From playlist Number Theory

Lec 6 | MIT 6.046J / 18.410J Introduction to Algorithms (SMA 5503), Fall 2005
Lecture 06: Order Statistics, Median View the complete course at: http://ocw.mit.edu/6-046JF05 License: Creative Commons BY-NC-SA More information at http://ocw.mit.edu/terms More courses at http://ocw.mit.edu
From playlist MIT 6.046J / 18.410J Introduction to Algorithms (SMA 5503),

Frédéric Vivien : Algorithmes d’approximation - Partie 2
Résumé : Dans la deuxième partie de ce cours nous considérerons un problème lié, celui des algorithmes compétitifs. Dans le cadre de l'algorithmique « en-ligne », les caractéristiques d'une instance d'un problème ne sont découvertes qu'au fur et à mesure du traitement de l'instance (comme
From playlist Mathematical Aspects of Computer Science

Nexus Trimester - Qi Chen (The Chinese University of Hong Kong)
Partition-Symmetrical Entropy Functions Qi Chen (The Chinese University of Hong Kong) February 15, 2016 Abstract: Let N={1,…,n}. Let p={N1,…,Nt} be a t-partition of N. An entropy function h is called p-symmetrical if for all A, B⊂N, h(A)=h(B) whenever |A∩Ni|=|B∩Ni|, i=1,…,t. We prove that
From playlist Nexus Trimester - 2016 - Fundamental Inequalities and Lower Bounds Theme

The hardest "What comes next?" (Euler's pentagonal formula)
Looks like I just cannot do short videos anymore. Another long one :) In fact, a new record in terms of the slideshow: 547 slides! This video is about one or my all-time favourite theorems in math(s): Euler's amazing pentagonal number theorem, it's unexpected connection to a prime numbe
From playlist Recent videos

Lecture 4 - Elementary Data Structures
This is Lecture 4 of the CSE373 (Analysis of Algorithms) taught by Professor Steven Skiena [http://www.cs.sunysb.edu/~skiena/] at Stony Brook University in 1997. The lecture slides are available at: http://www.cs.sunysb.edu/~algorith/video-lectures/1997/lecture5.pdf
From playlist CSE373 - Analysis of Algorithms - 1997 SBU

Rogers-Ramanujan Identities | Part 1: Introduction to Integer Partitions
This is the first in a series of videos where we explore the Rogers-Ramanujan identities. In this video we introduce the notion of an integer partition as well as give some examples and numerical evidence for certain identities. http://www.michael-penn.net http://www.randolphcollege.edu/m
From playlist Rogers-Ramanujan Identities

