
I is a Maximal Ideal iff R/I is a Field Proof
Please Subscribe here, thank you!!! https://goo.gl/JQ8Nys I is a Maximal Ideal iff R/I is a Field Proof
From playlist Abstract Algebra

Abstract Algebra | Maximal and prime ideals.
We prove some classic results involving maximal and prime ideals. Specifically we prove the an ideal P is prime iff R/P is an integral domain. Further, we prove that an ideal M is maximal iff R/M is a field. http://www.michael-penn.net https://www.researchgate.net/profile/Michael_Penn5 ht
From playlist Abstract Algebra

Rings 6 Prime and maximal ideals
This lecture is part of an online course on rings and modules. We discuss prime and maximal ideals of a (commutative) ring, use them to construct the spectrum of a ring, and give a few examples. For the other lectures in the course see https://www.youtube.com/playlist?list=PL8yHsr3EFj5
From playlist Rings and modules
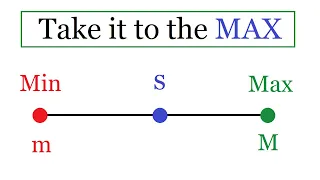
Maximum and Minimum of a set In this video, I define the maximum and minimum of a set, and show that they don't always exist. Enjoy! Check out my Real Numbers Playlist: https://www.youtube.com/playlist?list=PLJb1qAQIrmmCZggpJZvUXnUzaw7fHCtoh
From playlist Real Numbers

Proof: Prime Ideal iff R/P is Integral Domain; Maximal iff R/M is Field
A very useful theorem in ring theory is the theorem that an ideal P is prime if and only if the quotient R/P is an integral domain (ID). Similarly, an ideal M is maximal if and only if R/M is a field. In this video, we prove both of these statements! Ring & Module Theory playlist: https:/
From playlist Ring & Module Theory
Proof: Prime Ideals are Maximal in a PID
In a principal ideal domain, if an ideal is a prime ideal, that implies it is a maximal ideal, as long as it is not just the zero ideal. Here we give a straightforward explanation of this theorem from ring theory! Ring & Module Theory playlist: https://www.youtube.com/playlist?list=PLug5Z
From playlist Ring & Module Theory
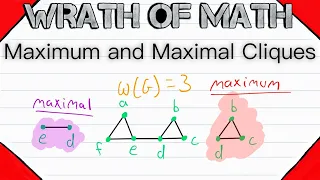
Maximum and Maximal Cliques | Graph Theory, Clique Number
What are maximum cliques and maximal cliques in graph theory? We'll be defining both terms in today's video graph theory lesson, as well as going over an example of finding maximal and maximum cliques in a graph. These two terms can be a little confusing, so let's dig in and clarify our un
From playlist Graph Theory

Every Nonzero Prime Ideal in a Principal Ideal Domain is Maximal Proof
Please Subscribe here, thank you!!! https://goo.gl/JQ8Nys Every Nonzero Prime Ideal in a Principal Ideal Domain is Maximal Proof
From playlist Abstract Algebra

When do fractional differential equations have maximal solutions?
When do fractional differential equations have maximal solutions? This video discusses this question in the following way. Firstly, a comparison theorem is formulated that involves fractional differential inequalities. Secondly, a sequence of approximative problems involving polynomials
From playlist Research in Mathematics

Commutative algebra 25 Artinian rings
This lecture is part of an online course on commutative algebra, following the book "Commutative algebra with a view toward algebraic geometry" by David Eisenbud. In this lecture we show that Artinian rings are Noetherian, probably the trickiest result of the course. As an application we
From playlist Commutative algebra

Commutative algebra 11 (Spectrum of a ring)
This lecture is part of an online course on commutative algebra, following the book "Commutative algebra with a view toward algebraic geometry" by David Eisenbud. In this lecture we define the spectrum of a ring as the space of prime ideals, and give a few examples. Reading: Lectures 9
From playlist Commutative algebra

Schemes 5: Definition of a scheme
This lecture is part of an online algebraic geometry course on schemes, based on chapter II of "Algebraic geometry" by Hartshorne. We give some historical background, then give the definition of a scheme and some simple examples, and finish by explaining the origin of the word "spectrum".
From playlist Algebraic geometry II: Schemes

Commutative algebra 32 Zariski's lemma
This lecture is part of an online course on commutative algebra, following the book "Commutative algebra with a view toward algebraic geometry" by David Eisenbud. We state and prove Zariski's lemma: Any field that is a finitely generated algebra over a field is a finitely generated modu
From playlist Commutative algebra

RNT2.1. Maximal Ideals and Fields
Ring Theory: We now consider special types of rings. In this part, we define maximal ideals and explore their relation to fields. In addition, we note three ways to construct fields.
From playlist Abstract Algebra

Visual Group Theory, Lecture 7.3: Ring homomorphisms
Visual Group Theory, Lecture 7.3: Ring homomorphisms A ring homomorphism is a structure preserving map between rings, which means that f(x+y)=f(x)+f(y) and f(xy)=f(x)f(y) both must hold. The kernel is always a two-sided ideal. There are four isomorphism theorems for rings, which are compl
From playlist Visual Group Theory

Existence Of Maximal Ideals - Feb 05, 2021- Rings and Modules
In this video we show using the axiom of choice that rings have maximal ideals.
From playlist Course on Rings and Modules (Abstract Algebra 4) [Graduate Course]

Applications of analysis to fractional differential equations
I show how to apply theorems from analysis to fractional differential equations. The ideas feature the Arzela-Ascoli theorem and Weierstrass' approximation theorem, leading to a new approach for solvability of certain fractional differential equations. When do fractional differential equ
From playlist Mathematical analysis and applications

Cayley Hamilton - April 09 2021
This is for my abstract algebra 4 course.
From playlist Course on Rings and Modules (Abstract Algebra 4) [Graduate Course]