
Interval of Convergence (silent)
Finding the interval of convergence for power series
From playlist 242 spring 2012 exam 3

The Difference Between Pointwise Convergence and Uniform Convergence
Please Subscribe here, thank you!!! https://goo.gl/JQ8Nys The Difference Between Pointwise Convergence and Uniform Convergence
From playlist Advanced Calculus

Math 131 111416 Sequences of Functions: Pointwise and Uniform Convergence
Definition of pointwise convergence. Examples, nonexamples. Pointwise convergence does not preserve continuity, differentiability, or integrability, or commute with differentiation or integration. Uniform convergence. Cauchy criterion for uniform convergence. Weierstrass M-test to imp
From playlist Course 7: (Rudin's) Principles of Mathematical Analysis

Find the Interval of Convergence
How to find the interval of convergence for a power series using the root test.
From playlist Convergence (Calculus)

Convergence: When ideas cross to produce something greater than the sum of it’s parts. We’re seeing this with fitness, travel, entertainment and the list goes on. Different ideas, different paths of research and development and different products are converging all around us. And where bet
From playlist CES 2016
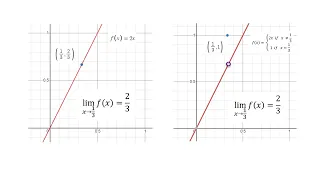
Calculus: How Convergence Explains The Limit
The limit definition uses the idea of convergence twice (in two slightly different ways). Once the of convergence is grasped, the limit concept becomes easy, even trivial. This clip explains convergence and shows how it can be used to under the limit.
From playlist Summer of Math Exposition Youtube Videos
Convergent sequences are bounded
Convergent Sequences are Bounded In this video, I show that if a sequence is convergent, then it must be bounded, that is some part of it doesn't go to infinity. This is an important result that is used over and over again in analysis. Enjoy! Other examples of limits can be seen in the
From playlist Sequences

What Does It Mean For A Series To Converge?
Please Subscribe here, thank you!!! https://goo.gl/JQ8Nys What Does It Mean For A Series To Converge? A series convergences to S if the sequence of partial sums converges to S. In this video I try to explain it and give an example. The example given is a version of Zeno's Dichotomy Paradox
From playlist Calculus 2 Exam 4 Playlist

Math 031 040317 Non-nonnegative Series: absolute convergence, Leibniz's test
[Sorry, no sound: the microphone was off.] Convergence test practice - which test would you use? Introduction to arbitrary series (i.e., not necessarily non-negative). Crude tool: absolute convergence implies convergence. Definition of absolute convergence. Conditional convergence. Le
From playlist Course 3: Calculus II (Spring 2017)

R. Perales - Recent Intrinsic Flat Convergence Theorems
Given a closed and oriented manifold M and Riemannian tensors g0, g1, ... on M that satisfy g0 gj, vol(M, gj)→vol (M, g0) and diam(M, gj)≤D we will see that (M, gj) converges to (M, g0) in the intrinsic flat sense. We also generalize this to the non-empty bundary setting. We remark that u
From playlist Ecole d'été 2021 - Curvature Constraints and Spaces of Metrics

R. Perales - Recent Intrinsic Flat Convergence Theorems (version temporaire)
Given a closed and oriented manifold M and Riemannian tensors g0, g1, ... on M that satisfy g0 gj, vol(M, gj)→vol (M, g0) and diam(M, gj)≤D we will see that (M, gj) converges to (M, g0) in the intrinsic flat sense. We also generalize this to the non-empty bundary setting. We remark that u
From playlist Ecole d'été 2021 - Curvature Constraints and Spaces of Metrics
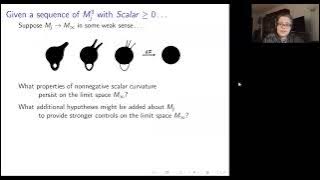
Christina Sormani - Sequences of manifolds with lower bounds on their scalar curvature
If one has a weakly converging sequence of manifolds with a uniform lower bound on their scalar curvature, what properties of scalar curvature persist on the limit space? What additional hypotheses might be added to provide stronger controls on the limit space? What hypotheses are requ
From playlist Not Only Scalar Curvature Seminar

Christina Sormani: A Course on Intrinsic Flat Convergence part 5
The lecture was held within the framework of the Hausdorff Trimester Program: Optimal Transportation and the Workshop: Winter School & Workshop: New developments in Optimal Transport, Geometry and Analysis
From playlist HIM Lectures 2015
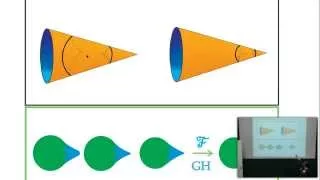
Christina Sormani: A Course on Intrinsic Flat Convergence part 1
Intrinsic Flat Convergence was first introduced in joint work with Stefan Wenger building upon work of Ambrosio-Kirchheim to address a question proposed by Tom Ilmanen. In this talk, I will present an overview of the initial paper on the topic [JDG 2011]. I will briefly describe key examp
From playlist HIM Lectures 2015

C. Sormani - Intrinsic Flat and Gromov-Hausdorff Convergence 4 (version temporaire)
We introduce various notions of convergence of Riemannian manifolds and metric spaces. We then survey results and open questions concerning the limits of sequences of Riemannian manifolds with uniform lower bounds on their scalar curvature. We close the course by presenting methods and the
From playlist Ecole d'été 2021 - Curvature Constraints and Spaces of Metrics
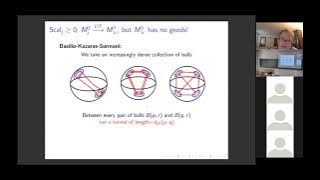
C. Sormani - Intrinsic Flat and Gromov-Hausdorff Convergence 2 (version temporaire)
We introduce various notions of convergence of Riemannian manifolds and metric spaces. We then survey results and open questions concerning the limits of sequences of Riemannian manifolds with uniform lower bounds on their scalar curvature. We close the course by presenting methods and the
From playlist Ecole d'été 2021 - Curvature Constraints and Spaces of Metrics

C. Sormani - Intrinsic Flat and Gromov-Hausdorff Convergence 4
We introduce various notions of convergence of Riemannian manifolds and metric spaces. We then survey results and open questions concerning the limits of sequences of Riemannian manifolds with uniform lower bounds on their scalar curvature. We close the course by presenting methods and the
From playlist Ecole d'été 2021 - Curvature Constraints and Spaces of Metrics
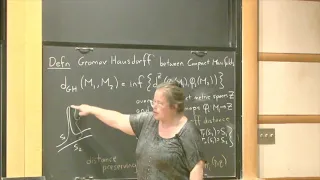
Introduction to Scalar Curvature and Convergence - Christina Sormani
Emerging Topics Working Group Topic: Introduction to Scalar Curvature and Convergence Speaker: Christina Sormani Affilaition: IAS Date: October 15, 2018 For more video please visit http://video.ias.edu
From playlist Mathematics

C. Sormani - Intrinsic Flat and Gromov-Hausdorff Convergence 2
We introduce various notions of convergence of Riemannian manifolds and metric spaces. We then survey results and open questions concerning the limits of sequences of Riemannian manifolds with uniform lower bounds on their scalar curvature. We close the course by presenting methods and the
From playlist Ecole d'été 2021 - Curvature Constraints and Spaces of Metrics

Newton's Method Interval of Convergence
How to find the Interval of Convergence for Newton-type methods such as Newton's Method, Secant Method, and Finite Difference Method including discussion on Damped Newton's Method and widening the convergence interval. Example code in R hosted on Github: https://github.com/osveliz/numerica
From playlist Root Finding