
What is the Riemann Hypothesis?
This video provides a basic introduction to the Riemann Hypothesis based on the the superb book 'Prime Obsession' by John Derbyshire. Along the way I look at convergent and divergent series, Euler's famous solution to the Basel problem, and the Riemann-Zeta function. Analytic continuation
From playlist Mathematics

A (compelling?) reason for the Riemann Hypothesis to be true #SOME2
A visual walkthrough of the Riemann Zeta function and a claim of a good reason for the truth of the Riemann Hypothesis. This is not a formal proof but I believe the line of argument could lead to a formal proof.
From playlist Summer of Math Exposition 2 videos

In this video, I prove two linear algebra facts with an almost identical proof! First, I show that every linear transformation from a finite dimensional vector space to itself must be bounded. Then I show that any two norms on a finite dimensional vector space are equivalent! YouTube chan
From playlist Linear Algebra
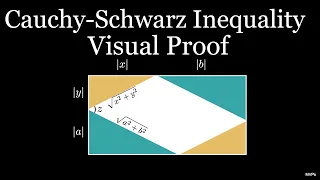
Cauchy Schwarz Inequality I (visual proof)
This is a short, animated visual proof of the two-dimensional Cauchy-Schwarz inequality (sometimes called Cauchy–Bunyakovsky–Schwarz inequality) using the Side-angle-side formula for the area of a parallelogram. #math #inequality #manim #animation #theorem #pww #proofwithoutwords #
From playlist Inequalities

Alan Turing and Number Theory - Yuri Matiyasevich (St. Petersburg) [2012]
slides for this talk: http://videolectures.net/site/normal_dl/tag=694395/turing100_matiyasevich_number_theory_01.pdf Alan Turing Centenary Conference Manchester, 2012 Alan Turing and Number Theory Yuri Matiyasevich, St.Petersburg Department of Steklov Mathematical Institute, Russian Aca
From playlist Mathematics
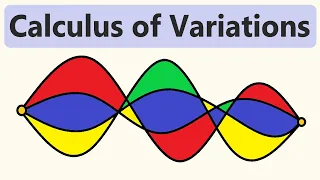
A Taste of Calculus of Variations
Dirichlet's Principle In this video, I give you a taste of calculus of variations by illustrating Dirichlet's principle, which says that a function u is a minimizer of a certain Dirichlet energy (kinetic + potential energy) if and only if u solves Poisson's equation. This is a neat way of
From playlist Partial Differential Equations

(ML 19.2) Existence of Gaussian processes
Statement of the theorem on existence of Gaussian processes, and an explanation of what it is saying.
From playlist Machine Learning

Solve a Bernoulli Differential Equation (Part 2)
This video provides an example of how to solve an Bernoulli Differential Equation. The solution is verified graphically. Library: http://mathispower4u.com
From playlist Bernoulli Differential Equations

B25 Example problem solving for a Bernoulli equation
See how to solve a Bernoulli equation.
From playlist Differential Equations
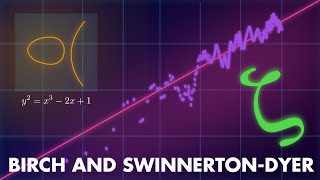
The Most Difficult Math Problem You've Never Heard Of - Birch and Swinnerton-Dyer Conjecture
The Birch and Swinnerton-Dyer Conjecture is a millennium prize problem, one of the famed seven placed by the Clay Mathematical Institute in the year 2000. As the only number-theoretic problem in the list apart from the Riemann Hypothesis, the BSD Conjecture has been haunting mathematicians
From playlist Math

Ptolemy's theorem and generalizations | Rational Geometry Math Foundations 131 | NJ Wildberger
The other famous classical theorem about cyclic quadrilaterals is due to the great Greek astronomer and mathematician, Claudius Ptolemy. Adopting a rational point of view, we need to rethink this theorem to state it in a purely algebraic way, without resort to `distances' and the correspon
From playlist Math Foundations
Solve a Bernoulli Differential Equation (Part 1)
This video provides an example of how to solve an Bernoulli Differential Equation. The solution is verified graphically. Library: http://mathispower4u.com
From playlist Bernoulli Differential Equations

Recent developments in non-commutative Iwasawa theory I - David Burns
David Burns March 25, 2011 For more videos, visit http://video.ias.edu
From playlist Mathematics

Giles Gardam: Kaplansky's conjectures
Talk by Giles Gardam in the Global Noncommutative Geometry Seminar (Americas) https://globalncgseminar.org/talks/3580/ on September 17, 2021.
From playlist Global Noncommutative Geometry Seminar (Americas)

Giles Gardam - Kaplansky's conjectures
Kaplansky made various related conjectures about group rings, especially for torsion-free groups. For example, the zero divisors conjecture predicts that if K is a field and G is a torsion-free group, then the group ring K[G] has no zero divisors. I will survey what is known about the conj
From playlist Talks of Mathematics Münster's reseachers

Gonçalo Tabuada - 1/3 Noncommutative Counterparts of Celebrated Conjectures
Some celebrated conjectures of Beilinson, Grothendieck, Kimura, Tate, Voevodsky, Weil, and others, play a key central role in algebraic geometry. Notwithstanding the effort of several generations of mathematicians, the proof of (the majority of) these conjectures remains illusive. The aim
From playlist Summer School 2020: Motivic, Equivariant and Non-commutative Homotopy Theory

Explicit formulae for Gross-Stark units and Hilbert’s 12th problem by Mahesh Kakde
PERFECTOID SPACES ORGANIZERS: Debargha Banerjee, Denis Benois, Chitrabhanu Chaudhuri, and Narasimha Kumar Cheraku DATE & TIME: 09 September 2019 to 20 September 2019 VENUE: Madhava Lecture Hall, ICTS, Bangalore Scientific committee: Jacques Tilouine (University of Paris, France) Eknath
From playlist Perfectoid Spaces 2019

Explicit formulae for Stark Units and Hilbert's 12th problem - Samit Dasgupta
Joint IAS/Princeton University Number Theory Seminar Topic: Explicit formulae for Stark Units and Hilbert's 12th problem Speaker: Samit Dasgupta Affiliation: Duke University Date: October 11, 2018 For more video please visit http://video.ias.edu
From playlist Mathematics

Gonçalo Tabuada - 3/3 Noncommutative Counterparts of Celebrated Conjectures
Some celebrated conjectures of Beilinson, Grothendieck, Kimura, Tate, Voevodsky, Weil, and others, play a key central role in algebraic geometry. Notwithstanding the effort of several generations of mathematicians, the proof of (the majority of) these conjectures remains illusive. The aim
From playlist Summer School 2020: Motivic, Equivariant and Non-commutative Homotopy Theory

Monotonicity of the Riemann zeta function and related functions - P Zvengrowski [2012]
General Mathematics Seminar of the St. Petersburg Division of Steklov Institute of Mathematics, Russian Academy of Sciences May 17, 2012 14:00, St. Petersburg, POMI, room 311 (27 Fontanka) Monotonicity of the Riemann zeta function and related functions P. Zvengrowski University o
From playlist Number Theory